 Short Answer Type
Short Answer TypeFind points on the curveВ ![]() В at which the tangents areВ (i) parallel to the x-axisВ (ii) parallel to the y-axis.
В at which the tangents areВ (i) parallel to the x-axisВ (ii) parallel to the y-axis.
 Long Answer Type
Long Answer TypeFind points on the curveВ ![]() В at which the tangents areВ (i) parallel to the x-axisВ (ii) parallel to the y-axis.
В at which the tangents areВ (i) parallel to the x-axisВ (ii) parallel to the y-axis.
For the curve y = 4x3 – 2x5, find all the points at which the tangent passes through the origin.
 Multiple Choice Questions
Multiple Choice Questions Long Answer Type
Long Answer TypeIf the curveВ О±x2В + ОІy2В = 1 and О±' x2В + ОІ'y2В = 1 intersect orthogonally, prove that (О± – О±') ОІ ОІ') = (ОІ – ОІ') О± О±'.В
The equations of two curves are
В В В В В В В В В В В В В В В ![]() В В В В В В В В В В В В В В В В В В В В ...(1)
В В В В В В В В В В В В В В В В В В В В ...(1)
and В В В В В В В В В ![]() В В В В В В В В В В В В В В В В В В В В В ...(2)
В В В В В В В В В В В В В В В В В В В В В ...(2)
Let curves (1) and (2) intersect atВ ![]()
![]() В В В В В В В В В В В В В В В В В В В В В В В В В В ...(3)
В В В В В В В В В В В В В В В В В В В В В В В В В В ...(3)
and В В В В В ![]() В В В В В В В В В В В В В В В В В В В В В В В В ...(4)
В В В В В В В В В В В В В В В В В В В В В В В В ...(4)
Subtracting (4) from (3), we get,
В В В В В В В В В В В В В В ![]()
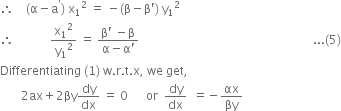
AtВ ![]()
Similarly for second curve,
В В В В В В В В В В В В В В В ![]()
Since the two curves (1) and (2) intersect orthogonally,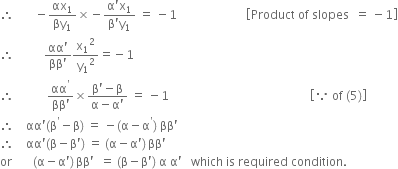
В В В В В
 Multiple Choice Questions
Multiple Choice Questions