Previous Year Papers

Download Solved Question Papers Free for Offline Practice and view Solutions Online.

Test Series

Take Zigya Full and Sectional Test Series. Time it out for real assessment and get your results instantly.

Test Yourself

Practice and master your preparation for a specific topic or chapter. Check you scores at the end of the test.

Application of Derivatives

Name: Zigya - For The Curious Learner
Rating: 4.4 (740 reviews)

Multiple Choice Questions

201.

The interval in which y = x²e^–x is increasing is

(– ∞, ∞)
(– 2, 0)
(2, ∞)
(2, ∞)

80 Views

Short Answer Type

202.
Find the maximum and the minimum values, if there be any, of f given by f(x) = 9x²– 6x + 1, x ∊ R.

The given function is
f (x) = 9x²– 6x + 1, x ∊ R
= (3x – 1) 2 ≥ 0, ∀ x ∊ R.
Also, f(x) = 0, if $straight x space equals space 1 third.$ Therefore, the minimum value of f is 0 and the point of minimum value of f is Futher,f has no maximum value and hence no point of maximum value of in R.

89 Views

203. Find the maximum and the minimum values of f, if there be any, given by
f(x) = – x², x ∊ R

87 Views

204.

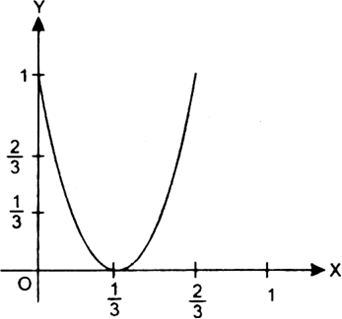
Find the maximum and the minimum values, if there be any, of f given by f(x) = x, x ∊ (0, 1).

83 Views

205.

Without using derivatives, find the maximum or minimum value of the function 9x²+ 12x + 2.

77 Views

206.

Find the maximum and minimum values, if any, of the following function without using the derivatives:
9x² – 12x + 4

97 Views

207.

Find the maximum and minimum values, if any, of the following function without using the derivatives:
4x ²+ 28x + 49

85 Views

208.

Find the maximum and minimum values, if any, of the following function without using the derivatives:
x + 1, x ∊ (– 1, 1)

92 Views

209.

Find the maximum and minimum values, if any, of the following functions without using the derivatives:
x²

89 Views

210.

Find the maximum and minimum values, if any, of the following functions without using the derivatives:
– (x – 2 )² + 4

78 Views