 Short Answer Type
Short Answer TypeGiven: ABC is an equilateral triangle. O is any point in the interior of the triangle. Perpendiculars OD, OE and OF are drawn from 0 on the sides BC, AB and AC respectively of ΔABC.
To Prove: OD + OE + OF = constant
Construction: Join O to A, B and C. Draw AH
⊥ BC.
Proof: ∵ ΔABC is an equilateral triangle AB = BC = CA![]()


Adding (1), (2) and (3), we get
⇐ OD + OE + OF = AH which is constant for a given triangle.
 Long Answer Type
Long Answer TypeABCD is a parallelogram whose diagonals intersect at O. If P is any point on BO, prove that
(i) ar(ΔADO) = ar(ΔCDO)
(ii) ar(ΔABP) = ar(ΔCBP)
 Short Answer Type
Short Answer Type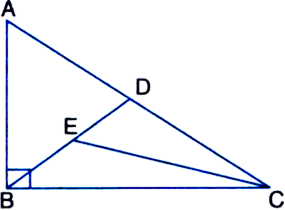
 Long Answer Type
Long Answer Type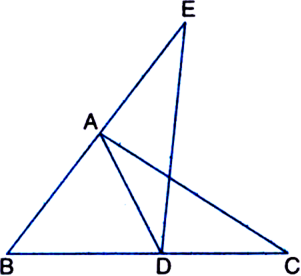
 Short Answer Type
Short Answer Type