 Short Answer Type
Short Answer Type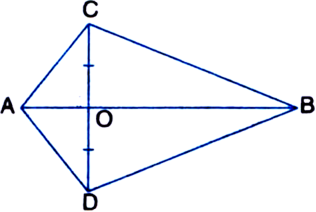
 Long Answer Type
Long Answer TypeABCD is a parallelogram whose diagonals intersect at O. If P is any point on BO, prove that
(i) ar(ΔADO) = ar(ΔCDO)
(ii) ar(ΔABP) = ar(ΔCBP)
 Short Answer Type
Short Answer Type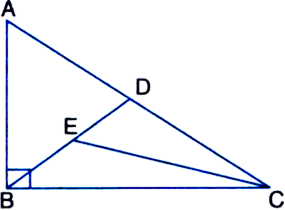
In right triangle ABC,
AB2 + BC2 = AC2
| By Pythagoras theorem ⇒ 62 + BC2 = 102
⇒ BC = 8 cm
∵ BD is a median of ΔABC
∵ A median of a triangle divides it into two triangles of equal areas ⇒ ar(ΔBCD) = 12 cm2 ...(2)
∵ E is the mid-point of BD ∴ CE is a median of ΔBCD![]()
∵ A median of a triangle divides it into two triangles of equal areas
 Long Answer Type
Long Answer Type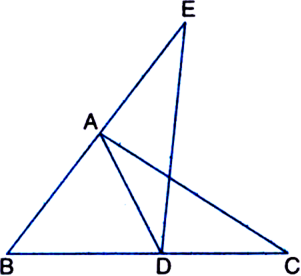
 Short Answer Type
Short Answer Type