 Short Answer Type
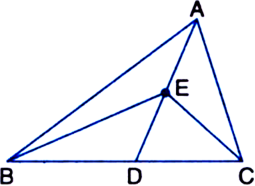
Short Answer Type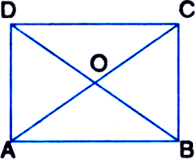
In figure, D and E are two points on BC such that BD = DE = EC. Show that ar(ΔABD) = ar(ΔADE) = ar(ΔAEC). [CBSE 2012 (March)]
Can you now answer the question that you have left in the ‘Introduction’, of this chapter, whether the field of Budhia has been actually divided into three parts of equal area?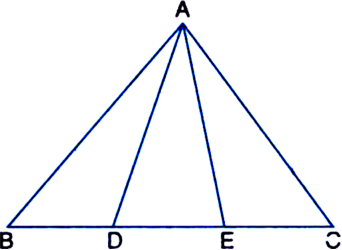
[Remark: Note that by taking BD = DE = EC, the triangle ABC is divided into three triangles ABD, ADE and AEC of equal areas. In the same way, by dividing BC into n equal parts and joining the points of division so obtained to the opposite vertex of BC, you can divide ΔABC into n triangles of equal areas.]

 Long Answer Type
Long Answer Type
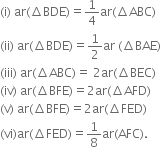
 Short Answer Type
Short Answer TypeDiagonals AC and BD of a quadrilateral ABCD intersect each other at P. Show that ar(ΔAPB) x ar(ΔCPD) = ar(ΔAPD) x ar(ΔBPC).
[Hint From A and C, draw perpendiculars to DD.]
 Long Answer Type
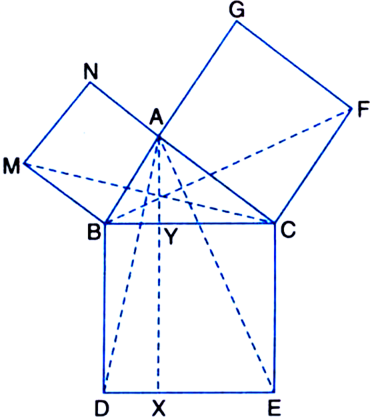
Long Answer Type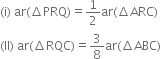
(i) ΔMBC ≅ ΔABD
(ii) ar(BYXD) = 2 ar(ΔMBC)
(iii) ar(BYXD) = ar(ΔABMN)
(iv) ΔFCB ≅ ΔACE
(v) ar(CYXE) = 2 ar(ΔFCB)
(vi) ar(CYXE) = ar(ACFG)
(vii) ar(BCED) = ar(ABMN) + ar(ACFG).
Note: Result (vii) is the famous Theorem of Pythagoras. You shall learn a simpler proof of this theorem in Class X.
Given: In figure, ABC is a right angle triangle right angled at A. BCED, ACFG and ABMN are squares on the sides BC, CA and AB respectively. Line segment AX ⊥ DE meets BC at Y.
To Prove: (i) ΔMBC ≅ ΔABD (ii) ar(BYXD) = 2 ar(ΔMBC)
(iii) ar(BYXD) = ar(ABMN)
(iv) ΔFCB ≅ ΔACE
(v) ar(CYXE) = 2 ar(ΔFCB)
(vi) ar(CYXE) = ar(ACFG)
(vii) ar(BCED) = ar(ABMN) + ar(ACFG) Proof: (i) In ΔMBC and ΔABD,
MB = AB ...(1)
| Sides of a square BC = BD ...(2)
| Sides of a square ∠MBA = ∠CBD | Each = 90°
⇒ ∠MBA + ∠ABC = ∠CBD + ∠ABC
[adding ∠ABC to both sides] ⇒ ∠MBC = ∠ABD ...(3)
In view of (1), (2) and (3),
ΔMBC = ΔABD.
| By Congruence SAS Rule
(ii) ar(BYXD) = 2 ar(ΔABD)
⇒ ar(BYXD) = 2 ar(ΔMBC).
(iii) ar(BYXD) = 2ar(ΔABD) ar(ABMN) = 2ar(ΔMBC) = 2ar(ΔABD)
| From (i)
∴ ar(BYXD) = ar(ABMN).
(iv) In ΔFCB and ΔACE,
FC = AC | Sides of a square
CB = CE | Sides of a square
∠FCA = ∠BCE | Each = 90°
⇒ ∠FCA + ∠ACB = ∠BCE + ∠ACB
(Adding the same on both sides) ⇒ ∠FCB = ∠ACE ∴ ΔFCB ≅ ΔACE.
| SAS Congruence Rule
(v) ar(CYXE) = 2ar(ΔACE) = 2ar(ΔFCB)
| From (iv) ∵ ΔFCB ≅ ΔACE ∴ ar(ΔFCB) = ar(ΔACE) Congruent As have equal areas
(vi) ar(CYXE) = 2ar(ΔACE) = 2ar(ΔFCB) ar(ACFG) = 2ar(ΔFCB) ∴ ar(CYXE) = ar(ACFG).
(vii) ar(BCED) = ar(CYXE) + ar(BYXD)
= ar(ACFG) + ar(ABMN) = ar(ABMN) + ar(ACFG).
