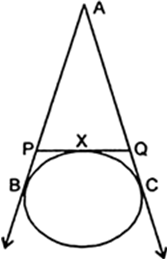
Short Answer Type
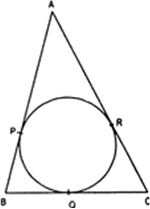
Short Answer TypeThe incircle of ΔABC touches the sides BC, CA and BA at D, E and F respectively. If
AB = AC, prove that BD = CD.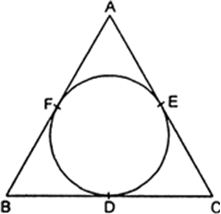
A quadrilateral ABCD is drawn to circumscribe a circle (Fig. 10.62). Prove that AB + CD = AD + BC.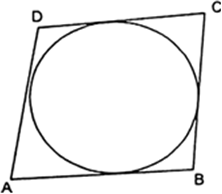
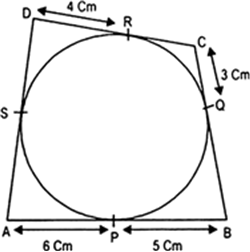

PT = PS
[Tangent from external points are equal]
⇒ PT = PB + BS
⇒ 12 = PB + BC [∴ BC = BS]
⇒ 12 = PB + 5
⇒ PB = 12 – 5 = 7 cm.