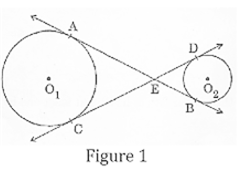
Short Answer TypeIn Figure 1, common tangents AB and CD to the two circles with centres O1 and O2 intersect at E. Prove that AB = CD.
The incircle of an isosceles triangle ABC, in which AB = AC, touches the sides BC, CA and AB at D, E and F respectively. Prove that BD = DC.
Long Answer TypeProve that the tangent at any point of a circle is perpendicular to the radius through the point of contact.
Prove that opposite sides of a quadrilateral circumscribing a circle subtend supplementary angles at the centre of the circle.
Short Answer TypeIf the angle between two tangents drawn from an external point P to a circle of radius a and centre O, is 60°, then find the length of OP.
A circle touches all the four sides of a quadrilateral ABCD. Prove that
AB + CD = BC + DA
Since tangents drawn from an external point to a circle are equal in length, we have
AP = AS ........(i)
BP = BQ ........(ii)
CR = CQ ........(iii)
DR = DS ........(iv)
Adding (i), (ii), (iii), (iv), we get
AP + BP + CR + DR = AS + BQ + CQ + DS
( AP + BP ) + ( CR + DR ) = ( AS + DS ) + ( BQ + CQ )
Prove that the tangents drawn at the end points of a chord of a circle make equal angles with the chord.
Long Answer TypeIn the given figure, XY and X’Y’ are two parallel tangents to a circle with centre O and another tangents AB with point of contact C, is intersecting XY at A and X’Y’ at B. Prove that ∠AOB = 90°.
Multiple Choice QuestionsIn Fig., the sides AB, BC and CA of a triangle ABC, touch a circle at P, Q and R respectively. If PA = 4 cm, BP = 3 cm and AC = 11 cm, then the length of BC (in cm) is
11
10
14
15
Switch
