 Short Answer Type
Short Answer TypeIn figure A,B and C are three points on a circle with centre O such that ∠ BOC = 30° and ∠ AOB = 60°. If D is a point on the circle other than the arc ABC, find ∠ADC.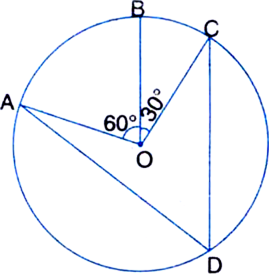
 Long Answer Type
Long Answer Type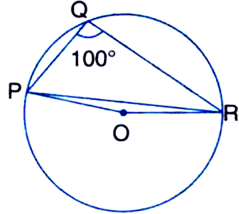
 Short Answer Type
Short Answer Type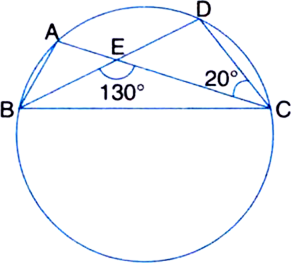
∠CED + ∠BEC = 180°
| Linear Pair Axiom
⇒ ∠CED + 130° = 180°
⇒ ∠CED = 180° - 130° = 50° ...(1)
∠ECD = 20° ...(2)
In ∆CED,
∠CED + ∠ECD + ∠CDE = 180°
| Sum of all the angles of a triangle is 180°
⇒ 50° + 20° + ∠CDE = 180°
| Using (1) and (2)
⇒ 70° + ∠CDE = 180°
⇒ ∠CDE = 180° - 70°
⇒ ∠CDE = 110° ...(3)
Now, ∠BAC = ∠CDE
| Angles in the same segment of a circle are equal
= 110°.
I Using (3).
 Long Answer Type
Long Answer TypeIf the non-parallel sides of a trapezium are equal, prove that it is cyclic. Prove that an isosceles trapezium is cyclic.
