 Short Answer Type
Short Answer Type
ABC and ADC are two right triangles with common hypotenuse AC. Prove that ∠ CAD = ∠ CBD.
 Long Answer Type
Long Answer Type
Given: In figure, a diameter AB of a circle bisects a chord PQ. AQ || PB.
To Prove: The chord PQ is also a diameter of the circle.
Proof: ∠AQP = ∠ABP ...(1)
| Angles in the same segment
∵ AQ || PB and QP intersects them
∴ ∠AQP = ∠QPB ...(2)
| Alt. Int. ∠s
From (1) and (2),
∠ABP = ∠QPB
⇒ ∠OBP = ∠OPB
∴ OP = OB ...(3)
| Sides opp. to equal angles
Again,
⇒ ∠BPQ = ∠BAQ ...(4)
| Angles in the same segment
∵ AQ || PB
and AB intersects them
∴ ∠BPQ = ∠PQA ...(5)
| Alt. Int. ∠s
From (4) and (5),
∠BAQ = ∠PQA
⇒ ∠OAQ = ∠OQA
∴ OQ = OA ...(6)
| Sides opp. to equal angles
Adding (3) and (6), we get
OP + OQ = OB + OA
⇒ PQ = AB
∵ AB is a diameter of the circle
∴ PQ is also a diameter of the circle.
 Short Answer Type
Short Answer Type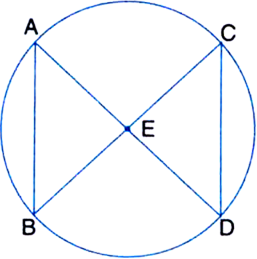
 Long Answer Type
Long Answer Type Short Answer Type
Short Answer Type