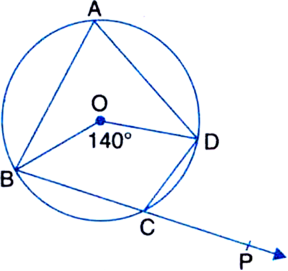
Long Answer Type
Long Answer Type
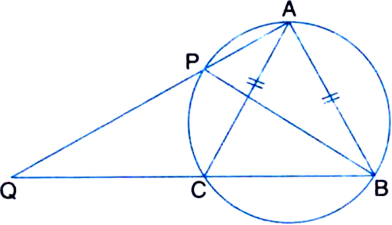
Given: ABC is a triangle and P is a point on the side BC such that AB = AP. AP produced meets the circumcircle of ∆ABC at Q.
To Prove: CP = CQ
Proof : ![]()
[ Angles in the same segment of a circle are equal ]
![]()
[ Vertically opposite angles ]![]()
[ AA criterion of similarily ]![]()
[ ![]() Corresponding sides of two similar triangles are proportional ]
Corresponding sides of two similar triangles are proportional ]![]()
But AB = AP | Given![]() CQ = CP
CQ = CP![]()
[ Angles opposite to equal sides of a triangle are equal ]
 Short Answer Type
Short Answer Type