6.

Construct the following angles and verify by measuring them by a protractor:

75°

(i) 75°

Given: A ray OA.
Required: To construct an angle of 75° at O.
Steps of Construction:
1. Taking O as centre and some radius, draw an arc of a circle, which intersects OA, say at a point B.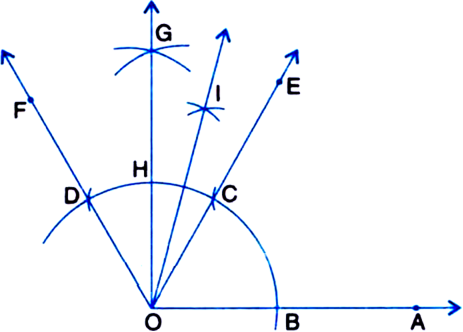

2. Taking B as centre and with the same radius as before, draw an arc intersecting the previously drawn arc, say at a point C.
3. Taking C as centre and with the same radius as before, draw an arc intersecting the arc drawn in step 1, say at D.
4. Join the ray OE passing through C. Then ∠EOA = 60°.
5. Draw the ray OF passing through D. Then ∠FOE = 60°.
6. Next, taking C and D as centres and with the radius more than  CD, draw arcs to intersect each other, say at G.
7. Draw the ray OG intersecting the arc of step 1 at H. This ray OG is the bisector of the intersect each other, say at G. 7. Draw the ray OG intersecting the arc of step 1 at H. This ray OG is the bisector of the angle FOE, 


8. Next, taking C and H as centres and with the radius more than  CH, draw arcs to intersect each other, say at I.
9. Draw the ray OI. This ray OI is the bisector of the angle GOE,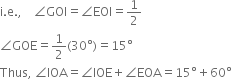 



On measuring the ∠IOA by protractor, we find that ∠IOA = 15°.
Thus the construction is verified.