9.

Construct an equilateral triangle, given its side and justify the construction.

Given: Side (say 6 cm) of an equilateral triangle.
Required: To construct the equilateral triangle and justify the construction.
Steps of Construction:
1. Take a ray AX with initial point A. From AX, cut off AB = 6 cm.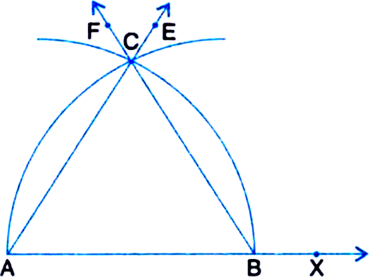



2. Taking A as centre and radius (= 6 cm), draw an arc of a circle, which intersects AX, say at a point B.
3. Taking B as centre and with the same radius as before, draw an arc intersecting the previously drawn arc, say at a point C.
4. Draw the ray AE passing through C.
5. Draw the ray BF passing through C. Then ∆ABC is the required triangle with given side 6 cm.
Justification:
AB = BC | By construction
AB = AC | By construction
∴ AB = BC = CA
∴ AABC is an equilateral triangle.
∴ The construction is justified.