Previous Year Papers

Download Solved Question Papers Free for Offline Practice and view Solutions Online.

Test Series

Take Zigya Full and Sectional Test Series. Time it out for real assessment and get your results instantly.

Test Yourself

Practice and master your preparation for a specific topic or chapter. Check you scores at the end of the test.

Continuity and Differentiability

Short Answer Type

271. Differentiate the following w.r.t. x :
x^x + x^a + a^x + a^a for some fixed a > 0 and x > 0

73 Views

272.

Find space dy over dx comma space if space straight y to the power of straight x plus straight x to the power of straight y plus straight x to the power of straight x equals straight a to the power of straight b.

74 Views

273. $If space straight y equals 1 plus fraction numerator straight c subscript 1 over denominator straight x minus straight c subscript 1 end fraction plus fraction numerator straight c subscript 2 straight x over denominator left parenthesis straight x minus straight c subscript 1 right parenthesis left parenthesis straight x minus straight c subscript 2 right parenthesis end fraction comma space prove space that space dy over dx equals straight y over straight x open parentheses fraction numerator straight c subscript 1 over denominator straight c subscript 1 minus straight x end fraction plus fraction numerator straight c subscript 2 over denominator straight c subscript 2 minus straight x end fraction close parentheses$

space space space straight y equals 1 plus fraction numerator straight c subscript 1 over denominator straight x minus straight c subscript 1 end fraction plus fraction numerator straight c subscript 2 straight x over denominator left parenthesis straight x minus straight c subscript 1 right parenthesis left parenthesis straight x minus straight c subscript 2 right parenthesis end fraction therefore space space straight y equals fraction numerator straight x minus straight c subscript 1 plus straight c subscript 1 over denominator straight x minus straight c subscript 1 end fraction plus fraction numerator straight c subscript 2 straight x over denominator left parenthesis straight x minus straight c subscript 1 right parenthesis left parenthesis straight x minus straight c subscript 2 right parenthesis end fraction rightwards double arrow space straight y equals fraction numerator straight x over denominator straight x minus straight c subscript 1 end fraction plus fraction numerator straight c subscript 2 straight x over denominator left parenthesis straight x minus straight c subscript 1 right parenthesis left parenthesis straight x minus straight c subscript 2 right parenthesis end fraction rightwards double arrow space fraction numerator straight x left parenthesis straight x minus straight c subscript 2 right parenthesis plus straight c subscript 2 straight x over denominator left parenthesis straight x minus straight c subscript 1 right parenthesis left parenthesis straight x minus straight c subscript 2 right parenthesis end fraction space rightwards double arrow space fraction numerator straight x squared minus xc subscript 2 plus straight c subscript 2 straight x over denominator left parenthesis straight x minus straight c subscript 1 right parenthesis left parenthesis straight x minus straight c subscript 2 right parenthesis end fraction rightwards double arrow space straight y equals fraction numerator straight x squared over denominator left parenthesis straight x minus straight c subscript 1 right parenthesis left parenthesis straight x minus straight c subscript 2 right parenthesis end fraction therefore space log space straight y equals log open square brackets fraction numerator straight x squared over denominator left parenthesis straight x minus straight c subscript 1 right parenthesis left parenthesis straight x minus straight c subscript 2 right parenthesis end fraction close square brackets space rightwards double arrow space log space straight y equals log space straight x squared minus log left square bracket left parenthesis straight x minus straight c subscript 1 right parenthesis left parenthesis straight x minus straight c subscript 2 right parenthesis right square bracket rightwards double arrow space log space straight y equals 2 log space straight x minus log left parenthesis straight x minus straight c subscript 1 right parenthesis left parenthesis straight x minus straight c subscript 2 right parenthesis Differentiating space both space sides space straight w. straight r. straight t. straight x comma space space space space space space 1 over straight y dy over dx equals 2 over straight x minus fraction numerator 1 over denominator straight x minus straight c subscript 1 end fraction minus fraction numerator 1 over denominator straight x minus straight c subscript 2 end fraction rightwards double arrow space dy over dx equals straight y open square brackets 2 over straight x minus fraction numerator 1 over denominator straight x minus straight c subscript 1 end fraction minus fraction numerator 1 over denominator straight x minus straight c subscript 2 end fraction close square brackets space rightwards double arrow space dy over dx equals straight y over straight x open square brackets 2 minus fraction numerator straight x over denominator straight x minus straight c subscript 1 end fraction minus fraction numerator straight x over denominator straight x minus straight c subscript 2 end fraction close square brackets rightwards double arrow space dy over dx equals straight y over straight x open square brackets open parentheses 1 minus fraction numerator 1 over denominator straight x minus straight c subscript 1 end fraction close parentheses plus open parentheses 1 minus fraction numerator 1 over denominator straight x minus straight c subscript 2 end fraction close parentheses close square brackets space rightwards double arrow space dy over dx equals straight y over straight x open square brackets fraction numerator straight x minus straight c subscript 1 minus straight x over denominator straight x minus straight c subscript 1 end fraction plus fraction numerator straight x minus straight c subscript 2 minus straight x over denominator straight x minus straight c subscript 2 end fraction close square brackets rightwards double arrow space dy over dx equals straight y over straight x open square brackets negative fraction numerator straight c subscript 1 over denominator straight x minus straight c subscript 1 end fraction minus fraction numerator straight c subscript 2 over denominator straight x minus straight c subscript 2 end fraction close square brackets space rightwards double arrow space dy over dx equals straight y over straight x open square brackets fraction numerator straight c subscript 1 over denominator straight c subscript 1 minus straight x end fraction plus fraction numerator straight c subscript 2 over denominator straight c subscript 2 minus straight x end fraction close square brackets

75 Views

274.

If space straight y equals fraction numerator ax over denominator left parenthesis straight x minus straight a right parenthesis left parenthesis straight x minus straight b right parenthesis left parenthesis straight x minus straight c right parenthesis end fraction plus fraction numerator bx over denominator left parenthesis straight x minus straight b right parenthesis left parenthesis straight x minus straight c right parenthesis end fraction plus fraction numerator straight c over denominator straight x minus straight c end fraction plus 1. prove space that space fraction numerator straight y apostrophe over denominator straight y end fraction equals 1 over straight x open parentheses fraction numerator straight a over denominator straight a minus straight x end fraction plus fraction numerator straight b over denominator straight b minus straight x end fraction plus fraction numerator straight c over denominator straight c minus straight x end fraction close parentheses

71 Views

275. If u, v, w are differentiable function ofx, then show that

straight d over dx left parenthesis straight u. straight v. straight w right parenthesis equals du over dx. vw plus straight u. dv over dx. straight w plus straight u. straight v dw over dx

in two ways - first by repeated application of product rule, second by logarithmic differentiation.

76 Views

276.

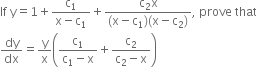
Differentiate (x²-5x+8)(x³+7x+ 9) in three ways mentioned below :
(i) by using product rule.
(ii) by expanding the product to obtain a single polynomial.
(iii) by logarithmic differentiation.
Also verify that three answers so obtained are the same.

72 Views