 Short Answer Type
Short Answer TypeLet f (x) = (x2Â - 1) (x - 2) = x3Â - 2 x2Â - x + 2
It is a polynomial in x
(a) Â Â Â Since every polynomial in x is a continuous function for every value of x.
∴ f(x) is continuous in [-1, 2].
(b) Â Â Â f'(x) = 3 x2Â - 4 x - 1, which exists in (-1, 2)
∴ f is derivable in (-1, 2).
(c) Â Â Â f(-1) = (1 - 1) (-1 -2) = 0
f(2) = (4 - 1) (2 - 1) (2 - 2) = 0
∴ f(-1) = f(2)
∴ f satisfies all the conditions of Rolle's Theorem
∴ there must exist at least one value c ofx such that f'(c) = 0 where - 1 < c < 2.
Now f'(c) = 0 gives us 3 c2Â - 4 c - 1 = 0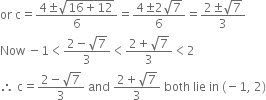
∴ Rolle's Theorem is verified.
