 Short Answer Type
Short Answer TypeFind the values of y for which the distance between the points P(2, – 3) and Q(10, y) is 10 units.
If Q(0, 1) is equidistant from P(5, –3) and R(x, 6), find the values of x. Also find the distances QR and PR.
Find the coordinates of the point which divides the join of (–1, 7) and (4, –3) in the ratio 2 : 3.
 Long Answer Type
Long Answer TypeLet P and Q be the point of trisection of AB. Then,
AP = PQ = QB = 1
Case I. Here P divides AB in the ratio 1 : 2.
So, we have
x1 = 4, y1 = -1
x2 = -2, y2 = -3
and m1 = 1, m2 = 2
∴ The coordinates of P are given by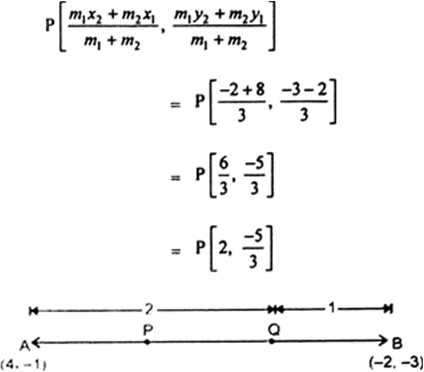
Case II. Here, Q divides AB in the ratio 2 : 1. So, we have
x1 = 4, y1 = -1
x2 = 2, y2 = -3
and m1 = 2, m2 = 1
∴ The coordinates of Q are given by
 Short Answer Type
Short Answer TypeTo conduct Sports Day activities, in your rectangular shaped school ground ABCD, lines have been drawn with chalk powder at a distance of 1m each. 100 flower pots have been placed at a distance of 1m from each other along AD, as shown in Fig. 7.12. Niharika runs ![]() th the distance AD on the 2nd line and posts a green flag. Preet runs
th the distance AD on the 2nd line and posts a green flag. Preet runs ![]() th the distance AD on the eighth line and posts a red flag. What is the distance between both the flags? If Rashmi has to post a blue flag exactly halfway between the line segment joining the two flags, where should she post her flag?
th the distance AD on the eighth line and posts a red flag. What is the distance between both the flags? If Rashmi has to post a blue flag exactly halfway between the line segment joining the two flags, where should she post her flag?
Fig. 7.12
Find the ratio in which the line segment joining the points (– 3, 10) and (6, – 8) is divided by (– 1, 6).
Find the ratio in which the line segment joining A(1, – 5) and B(– 4, 5) is divided by the x-axis. Also find the coordinates of the point of division
If (1, 2), (4, y), (x, 6) and (3, 5) are the vertices of a parallelogram taken in order, find x and y
