Previous Year Papers

Download Solved Question Papers Free for Offline Practice and view Solutions Online.

Test Series

Take Zigya Full and Sectional Test Series. Time it out for real assessment and get your results instantly.

Test Yourself

Practice and master your preparation for a specific topic or chapter. Check you scores at the end of the test.

Solve by matrix method:
$3 over straight x plus 4 over straight y plus 7 over straight z space equals space 14 2 over straight x minus 1 over straight y plus 3 over straight z space equals space 4 1 over straight x plus 2 over straight y minus 3 over straight z space equals space 0$

92 Views

302.

Solve the system of the following equations:
$2 over straight x plus 3 over straight y plus 10 over straight z space equals space 4 4 over straight x minus 6 over straight y plus 5 over straight z space equals space 1 6 over straight x plus 9 over straight y minus 20 over straight z space equals space 2$

75 Views

303.

If $straight A space equals space open square brackets table row 2 cell space space space minus 3 end cell cell space space space space 5 end cell row 3 cell space space space space space 2 end cell cell space minus 4 end cell row 1 cell space space space 1 end cell cell space minus 2 end cell end table close square brackets comma$ find A^-^1,Using A^-¹, solve the following system of linear equations.
2x – 3y + 5z = 16
3x + 2y – 4z = – 4
x + y – 2z = – 3

75 Views

304.

If $straight A space equals space open square brackets table row 2 cell space space space minus 3 end cell cell space space space space 5 end cell row 3 cell space space space space 2 end cell cell negative 4 end cell row 1 cell space space space 1 end cell cell negative 2 end cell end table close square brackets comma$ find A ^–1 .
Using A^–1, solve the following system of linear equations.
2x – 3y + 5z = 11
3x + 2y – 4z = – 5
x + y – 2z = – 3

74 Views

305.
Compute A^–1 for the following matrix
$straight A space equals space open square brackets table row cell negative 1 end cell cell space space space space space 2 end cell cell space space space space 5 end cell row cell space space 2 end cell cell space space minus 3 end cell cell space space space 1 end cell row cell negative 1 end cell cell space space 1 end cell cell space space space space 1 end cell end table close square brackets.$
Hence solve the system of equations.
– x + 2y+ 5 z = 2
2x – 3y + Z = 15
– x + y + z = – 3

Here $straight A space equals space open square brackets table row cell negative 1 end cell cell space space space space 2 end cell cell space space 5 end cell row 2 cell space minus 3 end cell cell space space 1 end cell row cell negative 1 end cell cell space space space space 1 end cell cell space space 1 end cell end table close square brackets$

$therefore space space space open vertical bar straight A close vertical bar space equals space open vertical bar table row cell negative 1 end cell cell space space space space 2 end cell cell space space space 5 end cell row 2 cell space minus 3 end cell cell space space 1 end cell row cell negative 1 end cell cell space space 1 end cell cell space space 1 end cell end table close vertical bar$

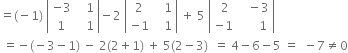
$equals left parenthesis negative 1 right parenthesis space open vertical bar table row cell negative 3 end cell cell space space space 1 end cell row 1 cell space space space 1 end cell end table close vertical bar minus 2 space open vertical bar table row 2 cell space space space 1 end cell row cell negative 1 end cell cell space space space 1 end cell end table close vertical bar space plus space 5 space open vertical bar table row cell space 2 end cell cell space space space minus 3 end cell row cell negative 1 end cell cell space space space space space space 1 end cell end table close vertical bar space equals negative left parenthesis negative 3 minus 1 right parenthesis space minus space 2 left parenthesis 2 plus 1 right parenthesis space plus space 5 left parenthesis 2 minus 3 right parenthesis space space equals space 4 minus 6 minus 5 space equals space space minus 7 not equal to 0$
Co-factors of the elements of the first row of | A | are
$open vertical bar table row cell negative 3 end cell cell space space space space 1 end cell row 1 cell space space space 1 end cell end table close vertical bar comma space space space minus open vertical bar table row cell space 2 end cell cell space space space space 1 end cell row cell negative 1 end cell cell space space space 1 end cell end table close vertical bar comma space space space space open vertical bar table row cell space space 2 end cell cell space space space minus 3 end cell row cell negative 1 end cell cell space space space space space space 1 end cell end table close vertical bar$
i.e., – 4, – 3, – 1 respectively
Co-factors of the elements of the second row of | A | are
$negative open vertical bar table row 2 cell space space space 5 end cell row 1 cell space space space 1 end cell end table close vertical bar comma space space space open vertical bar table row cell negative 1 end cell cell space space space 5 end cell row cell negative 1 end cell cell space space space 1 end cell end table close vertical bar comma space space minus open vertical bar table row cell negative 1 end cell cell space space space space 2 end cell row cell negative 1 end cell cell space space space space 1 end cell end table close vertical bar$

i.e., 3, 4, – 1 respectively
Co-factors of the elements of the third row of | A | are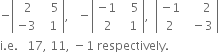
$negative open vertical bar table row cell space 2 end cell cell space space space 5 end cell row cell negative 3 end cell cell space space 1 end cell end table close vertical bar comma space space space minus open vertical bar table row cell negative 1 end cell cell space space space 5 end cell row cell space 2 end cell cell space space 1 end cell end table close vertical bar comma space space open vertical bar table row cell negative 1 end cell cell space space space space space 2 end cell row 2 cell space space minus 3 end cell end table close vertical bar straight i. straight e. space space space 17 comma space 11 comma space minus 1 space respectively.$
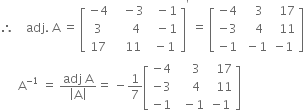
$therefore space space space space adj. space straight A space equals space open square brackets table row cell negative 4 end cell cell space space space minus 3 end cell cell space space minus 1 end cell row 3 cell space space space space space 4 end cell cell space space minus 1 end cell row 17 cell space space space 11 end cell cell space minus 1 end cell end table close square brackets to the power of apostrophe space equals space open square brackets table row cell negative 4 end cell cell space space space 3 end cell cell space space 17 end cell row cell negative 3 end cell cell space space space 4 end cell cell space space 11 end cell row cell negative 1 end cell cell space minus 1 end cell cell negative 1 end cell end table close square brackets space space space space space space straight A to the power of negative 1 end exponent space equals space fraction numerator adj space straight A over denominator open vertical bar straight A close vertical bar end fraction equals space minus 1 over 7 open square brackets table row cell negative 4 end cell cell space space space space 3 end cell cell space space 17 end cell row cell negative 3 end cell cell space space space space 4 end cell cell space space 11 end cell row cell negative 1 end cell cell space space minus 1 end cell cell negative 1 end cell end table close square brackets$

The given equations are
– r + 2y + 5z = 2
2x – 3y + z = 15
– x + y + z = – 3
These equations can be written as
$open square brackets table row cell negative 1 end cell cell space space space space space 2 end cell cell space space space 5 end cell row cell space space 2 end cell cell space minus 3 end cell cell space space space 1 end cell row cell negative 1 end cell cell space space space 1 end cell cell space space 1 end cell end table close square brackets space space open square brackets table row straight x row straight y row straight z end table close square brackets space equals space open square brackets table row cell space space 2 end cell row cell space space 15 end cell row cell negative 3 end cell end table close square brackets space space space space or space space space space AX space equals space straight B$

where $straight A space equals space open square brackets table row cell negative 1 end cell cell space space space space 2 end cell cell space space 5 space end cell row cell space space 2 end cell cell negative 3 end cell cell space 1 end cell row cell negative 1 end cell cell space space 1 end cell cell space 1 end cell end table close square brackets comma space space space space straight X space equals space open square brackets table row straight x row straight y row straight z end table close square brackets comma space space space straight B space equals space open square brackets table row cell space 2 end cell row 15 row cell negative 3 end cell end table close square brackets$

$therefore space space space space space space straight X space equals space straight A to the power of negative 1 end exponent straight B$

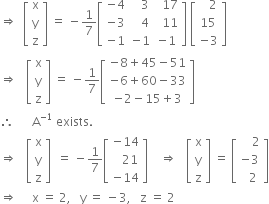
$rightwards double arrow space space open square brackets table row straight x row straight y row straight z end table close square brackets space equals space minus 1 over 7 open square brackets table row cell negative 4 end cell cell space space space 3 end cell cell space space 17 end cell row cell negative 3 end cell cell space space space 4 end cell cell space space 11 end cell row cell negative 1 end cell cell negative 1 end cell cell negative 1 end cell end table close square brackets space open square brackets table row cell space space space 2 end cell row 15 row cell negative 3 end cell end table close square brackets rightwards double arrow space space space open square brackets table row straight x row straight y row straight z end table close square brackets space equals space minus 1 over 7 open square brackets table row cell negative 8 plus 45 minus 51 end cell row cell negative 6 plus 60 minus 33 end cell row cell negative 2 minus 15 plus 3 end cell end table close square brackets therefore space space space space space space straight A to the power of negative 1 end exponent space exists. rightwards double arrow space space space open square brackets table row straight x row straight y row straight z end table close square brackets space space equals space minus 1 over 7 open square brackets table row cell negative 14 end cell row cell space space space 21 end cell row cell negative 14 end cell end table close square brackets space space space space rightwards double arrow space space space open square brackets table row straight x row straight y row straight z end table close square brackets space equals space open square brackets table row cell space space space space 2 end cell row cell negative 3 end cell row cell space space 2 end cell end table close square brackets rightwards double arrow space space space space space straight x space equals space 2 comma space space space straight y space equals space minus 3 comma space space space straight z space equals space 2$

78 Views

306.

Compute A^–1 for the following matrix
$straight A space equals space open square brackets table row 1 cell space space space space space 2 end cell cell space space space 5 end cell row 1 cell space minus 1 end cell cell space minus 1 end cell row 2 cell space space space 3 end cell cell space minus 2 end cell end table close square brackets.$
Hence, solve the system of equations:
x + 2y + 5z = 10
x – y – z = – 2
2x + 3y – 2z = – 1

97 Views

307.

If $straight A equals space open square brackets table row 3 cell space space space minus 2 end cell cell space space space space space 1 end cell row 2 cell space space space space 1 end cell cell space minus 3 end cell row cell negative 1 end cell cell space space space 2 end cell cell space space space 1 end cell end table close square brackets comma$ Find A^–1.

Using A solve the following systems of linear equations:
3x – 2y + z = 2
2y + y – 3z = – 5
– x + 2y + z = 6.

75 Views

Short Answer Type

308.

Investigate for what values of a and b the simultaneous equations:
x + y + z = 6
x + 2y + 3z = 10
x + 2y + az = b have a unique solution.

86 Views

Long Answer Type

309.

The sum of three numbers is 6. If we multiply third number by 3 and add second number to it, we get 11. By adding first and third numbers, we get double of the second number. Represent it algebraically and find the numbers using matrix method.

86 Views

310. The cost of 4 kg. onion, 3 kg. wheat and 2 kg. rice is Rs. 60. The cost of 2 kg. onion, 4 kg. wheat and 6 kg. rice is Rs. 90. The cost of 6 kg. onion. 2 kg. wheat and 3 kg. rice is Rs. 70. Find cost of each item per kg. by matrix method.

77 Views

Previous Year Papers

Test Series

Test Yourself

Long Answer Type

305. Compute A–1 for the following matrix Hence solve the system of equations.– x + 2y+ 5 z = 22x – 3y + Z = 15– x + y + z = – 3

Short Answer Type

Long Answer Type