5. Solve the following Linear Programming Problems graphically:
Minimise    Z = - 3x + 4 y
subject to the constraints: x + 2y ≤ 8, 3x + 2y ≤ 12, x ≥ 0, y ≥ 0

We are to minimise
Z = - 3x + 4 y
subject to the constraints
x + 2 y ≤ 8
3x + 2 y ≤ 12
x ≥ 0, y ≥ 0
Consider a set of rectangular cartesian axes OXY in the plane.
It is clear that any point which satisfies x ≥ 0, y ≥ 0 lies in the first quadrant.
Let us draw the graph of x + 2 y = 8
For x = 0, 2 y = 8 or y = 4
For y = 0, x = 8
∴  line meets OX in A(8, 0) and OY in L(0, 4).
Again we draw the graph of 3x + 2 y = 12
For x = 0, 2 y = 12 or y = 6
For y = 0, 3x = 12 or x = 4
∴  line meets OX in B(4, 0) and OY in M(0, 6).
Since feasible region is the region which satisfies all the constraints.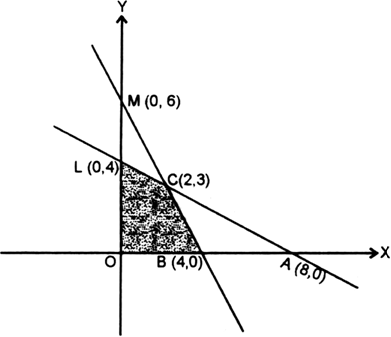

∴ OBCL is the feasible region and O(0, 0), B(4, 0), C(2, 3), L(0, 4) are comer points.
At O(0, 0), Z = 0 + 0 = 0
At B(4, 0), Z = -12 + 0 = -12
At C(2, 3), Z = -6 + 12 = 6
At L(0, 4), Z = 0 + 16 = 16
∴  minimum value = -12 at (4, 0).