Short Answer Type
Short Answer TypeIn figure, if PQ || ST, ∠PQR = 110° and ∠RST = 130°, find ∠QRS.
[Hint. Draw a line parallel to ST through point R.]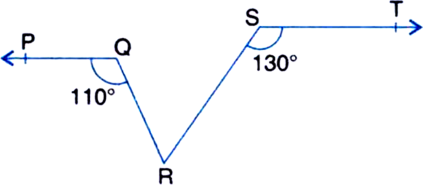
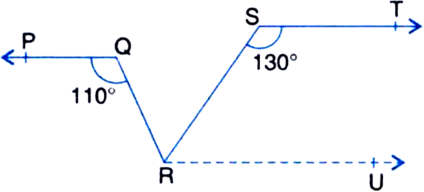
∠RST + ∠SRU = 180°
| Sum of the consecutive interior angles on the same side of the transversal is 180°
⇒ 130° + ∠SRU = 180°
⇒ ∠SRU = 180° - 130° = 50° ...(1)
∠QRU = ∠PQR = 110°
| Alternate Interior Angles
⇒ ∠QRS + ∠SRU = 110°
⇒ ∠QRS + 50° = 110° | Using (1)
⇒ ∠QRS = 110° - 50° = 60°.

In figure, EF is a transversal to two parallel lines AB and CD, GM and HL are the bisectors of the corresponding angles EGB and EHD. Prove that GM || HL.
[Hint. First prove that ∠EGM = ∠GHL]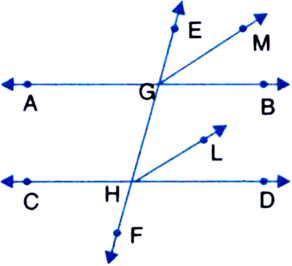
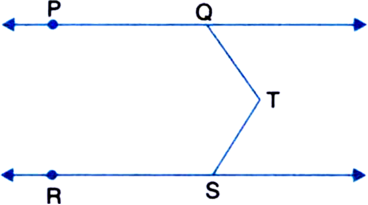
In figure, PQ || RS and T is any point as shown in the figure then show that
∠PQT + ∠QTS + ∠RST = 360°.