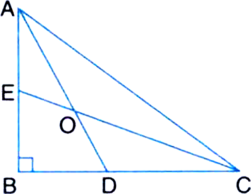
Short Answer Type
Short Answer Type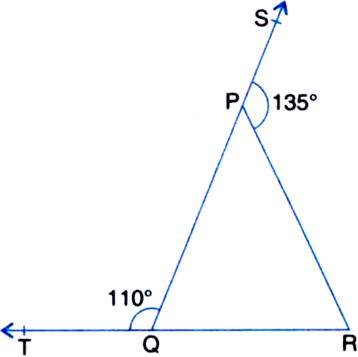
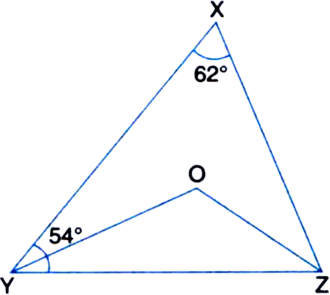
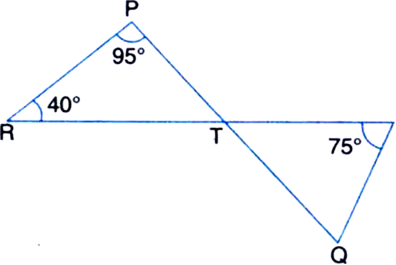
In ∆XYZ,
∠XYZ + ∠YZX + ∠ZXY = 180°
| ∵ The sum of all the angles of a triangle is 180°
⇒ 54° + ∠YZX + 62° = 180°
⇒ 116° + ∠YZX = 180°
⇒ ∠YZX = 180° - 116° = 64° ...(1)
∵ YO is the bisector of ∠XYZ
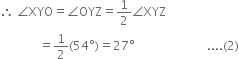
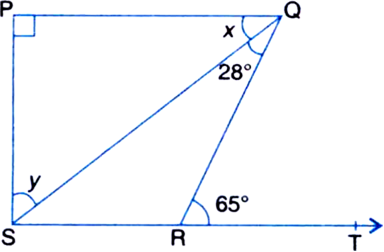
In ∆OYZ,
∠OYZ + ∠OZY + ∠YOZ = 180°
|∵ The sum of all the angles of a triangle is 180°
⇒ 27° + 32° + ∠YOZ = 180°
| Using (2) and (3)
⇒ 59° + ∠YOZ = 180°
⇒ ∠YOZ = 180° - 59° = 121°.
 Long Answer Type
Long Answer Type Short Answer Type
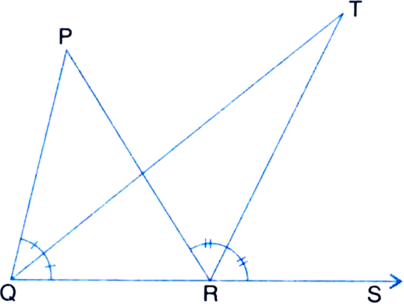
Short Answer TypeThe side EF, FD and DE of a triangle DEF are produced in order forming three exterior angles DFP, EDQ and FER respectively. Prove that
∠DFP + ∠EDQ + ∠FER = 360°.
OR
Prove that the sum of the exterior angles of a triangl is 360°.