Short Answer Type
Short Answer Type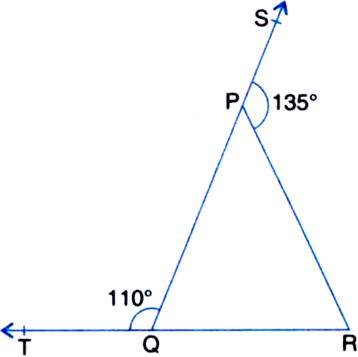
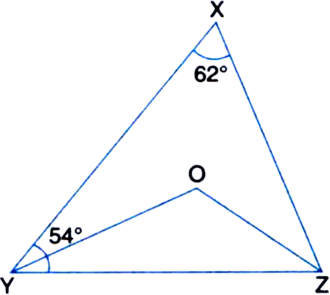
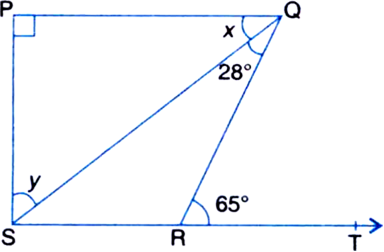
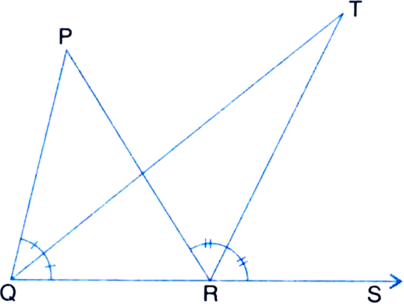
 Long Answer Type
Long Answer Type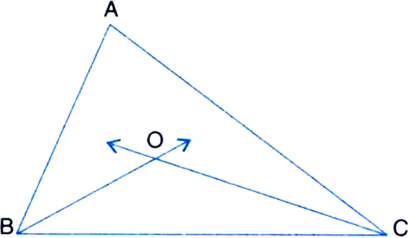
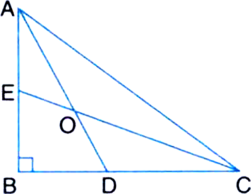
In ∆OBC, ∠BOC + ∠OBC + ∠OCB = 180°
| ∵ The sum of the three angles of a ∆ is 180°![]()
| From (1) and (2)![]()
In ∠ABC, ∠A + ∠B + ∠C = 180°
| ∵ The sum of the three angles of a triangle is 180°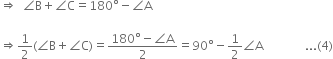
From (3) and (4), we have![]()
 Short Answer Type
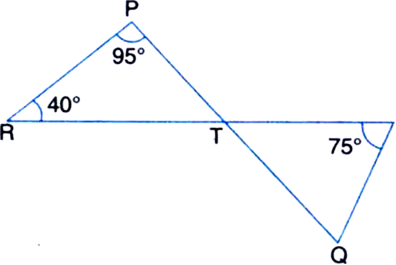
Short Answer TypeThe side EF, FD and DE of a triangle DEF are produced in order forming three exterior angles DFP, EDQ and FER respectively. Prove that
∠DFP + ∠EDQ + ∠FER = 360°.
OR
Prove that the sum of the exterior angles of a triangl is 360°.