Previous Year Papers

Download Solved Question Papers Free for Offline Practice and view Solutions Online.

Test Series

Take Zigya Full and Sectional Test Series. Time it out for real assessment and get your results instantly.

Test Yourself

Practice and master your preparation for a specific topic or chapter. Check you scores at the end of the test.

Practical Geometry

Short Answer Type

21. Can you construct the quadrilateral PLAN if PL = 6 cm, LA = 9.5 cm, ∠P = 75°, ∠L = 150° and ∠A = 140°? (Hint: Recall angle-sum property)

328 Views

22. In a parallelogram, the lengths of adjacent sides are known. Do we still need measures of the angles to construct as in the example above?

251 Views

23. Construct the following quadrilateral:
Quadrilateral DEAR
DE = 4 cm
EA = 5 cm
AR = 4.5 cm
∠E = 60°
∠A = 90°

884 Views

24. Construct the following quadrilateral:
Quadrilateral TRUE
TR = 3.5 cm
RU = 3 cm
UE = 4 cm
∠R = 75°
∠U = 120°

924 Views

25. Construct a quadrilateral ABCD, where AB = 4 cm, BC = 5 cm, CD = 6.5 cm and

angle straight B space equals space 105 degree

and

angle straight C space equals space 80 degree.

Here, we first drew BC. Instead, what could have been be the other starting points?

807 Views

26.

We used some five measurements to draw quadrilaterals so far. Can there be different sets of five measurements (other than seen so far) to draw a quadrilateral? The following problems may help you in answering the question.
(i) Quadrilateral ABCD with AB = 5 cm, BC = 5.5 cm, CD = 4 cm, AD = 6 cm and ∠B = 80°.
(ii) Quadrilateral PQRS with PQ = 4.5 cm, ∠P = 70°, ∠Q = 100°, ∠R = 80° and ∠S = 110°.
Construct a few more examples of your own to find sufficiency/insufficiency of the data for construction of a quadrilateral.

273 Views

27. How will you construct a rectangle PQRS if you know only the lengths PQ and QR?

657 Views

28.
Construct the kite EASY if AY = 8 cm, EY = 4 cm and SY = 6 cm (figure below) Which properties of the kite did you use in the process?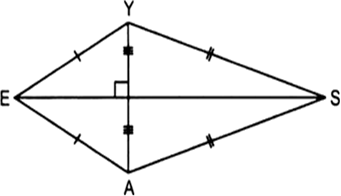

Following properties have been used in constructing the KITE:
(i) Diagonals are at right angles.
(ii) One of the diagonal bisects the other.
(iii) Pairs of consecutive sides are equal.

Steps of construction:
I. Draw a line segment AY = 8 cm.
II. Draw $space space space space PQ with rightwards arrow on top$ , the perpendicular bisector of AY such that it meets AY at O.
III. We cannot locate a point E on PQ at 4 cm from Y and A, i.e. EY = 4cm = EA is not possible. It is possible only When E and O coincide. In that case the kite does not exist.

Following properties have been used in constructing the KITE:(i) Diag