Previous Year Papers

Download Solved Question Papers Free for Offline Practice and view Solutions Online.

Test Series

Take Zigya Full and Sectional Test Series. Time it out for real assessment and get your results instantly.

Test Yourself

Practice and master your preparation for a specific topic or chapter. Check you scores at the end of the test.

Probability

Short Answer Type

1061. An unbiased coin is tossed six times. Find the probability of obtaining at least 2 heads.

81 Views

1062. In a box containing 100 bulbs, 10 are defective. What is the probabilities that out of a sample of 5 bulbs
(i) none is defective (ii) exactly two are defective.

106 Views

1063. If “getting a 6” in a throw of an unbiased die is a success and the random variable x denotes the number of successes in five throws of the die, find P (2 < x < 5)

92 Views

1064. If “getting a 5 or a 6” in the throw of an unbiased die is a success and the random variable x denotes the number of successes in 6 throws of the die, find P (x ≤ 4).

97 Views

Long Answer Type

1065. A die is thrown again and again until three sixes are obtained. Find the probability of obtaining the third six in the sixth throw of the dice.

286 Views

Short Answer Type

1066. Eight coins are thrown simultaneously. Find the probability of getting at least six heads.

436 Views

Long Answer Type

1067.
A pair of dice is thrown 7 times. If getting a total of 7 is considered a success, what is the probability of
(i) no success? (ii) 6 successes? (iii) at least 6 successes? (iv) at most 6 successes?

Here n = 7
Let p be the probability of getting a total of 7.

$therefore space space space space straight p space equals space 6 over 36 space equals 1 over 6 comma space space space straight q space equals space 1 minus 1 over 6 space equals 5 over 6$

(i) P(no success) = P(0) = $straight C presuperscript 7 subscript 0 space open parentheses 5 over 6 close parentheses to the power of 7 minus 0 end exponent space open parentheses 1 over 6 close parentheses to the power of 0 space equals space open parentheses 5 over 6 close parentheses to the power of 7$

(ii) P(6 successes) = P(6) = $straight C presuperscript 7 subscript 6 space open parentheses 5 over 6 close parentheses to the power of 7 minus 6 end exponent space open parentheses 1 over 6 close parentheses to the power of 6 space equals space 7 over 1 cross times 5 over 6 cross times open parentheses 1 over 6 close parentheses to the power of 6 space equals space 35 space open parentheses 1 over 6 close parentheses to the power of 7$

(iii) P(at least 6 (successes) = P(6) + P(7)

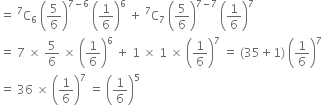
$equals space straight C presuperscript 7 subscript 6 space open parentheses 5 over 6 close parentheses to the power of 7 minus 6 end exponent space open parentheses 1 over 6 close parentheses to the power of 6 space plus space straight C presuperscript 7 subscript 7 space open parentheses 5 over 6 close parentheses to the power of 7 minus 7 end exponent space open parentheses 1 over 6 close parentheses to the power of 7 equals space 7 space cross times space 5 over 6 space cross times space open parentheses 1 over 6 close parentheses to the power of 6 space plus space 1 space cross times space 1 space cross times space open parentheses 1 over 6 close parentheses to the power of 7 space equals space left parenthesis 35 plus 1 right parenthesis space open parentheses 1 over 6 close parentheses to the power of 7 equals space 36 space cross times space open parentheses 1 over 6 close parentheses to the power of 7 space equals space open parentheses 1 over 6 close parentheses to the power of 5$
(iv) P(at most 6 successes) = P(not 7 success)
$equals space 1 minus straight P left parenthesis 7 right parenthesis space equals space 1 minus straight C presuperscript 7 subscript 7 space open parentheses 5 over 6 close parentheses to the power of 7 minus 7 end exponent space open parentheses 1 over 6 close parentheses to the power of 7 space equals space 1 minus open parentheses 1 over 6 close parentheses to the power of 7$