Short Answer Type
Short Answer TypeLet ABCD be a quadrilateral in which
∠A : ∠B : ∠C : ∠D = 3 : 5 : 9 : 13
Sum of the ratios = 3 + 5 + 9+ 13 = 30
Also, ∠A + ∠B + ∠C + ∠D = 360°
Sum of all the angles of a quadrilateral is 360°
 Long Answer Type
Long Answer Type Short Answer Type
Short Answer TypeDiagonal AC of a parallelogram ABCD bisects ∠A (see figure). Show that:
(i) it bisects ∠C also
(ii) ABCD is a rhombus.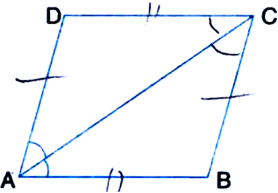
 Long Answer Type
Long Answer Type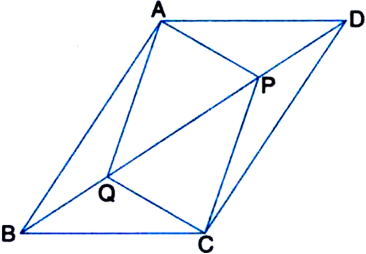
(i) ∆APD ≅ ∆CQB
(ii) AP = CQ
(iii) ∆AQB ≅ ∆CPD
(iv) AQ = CP
(v) APCQ is a parallelogram.
 Short Answer Type
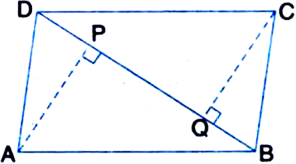
Short Answer TypeABCD is a parallelogram and AP and CQ are perpendiculars from vertices A and C on diagonal BD respectively (see figure). Show that:
(i) ∆APB ≅ ∆CQD
(ii) AP = CQ.