Short Answer TypeGiven: In parallelogram ABCD, AC = BD.
To Prove: ||gm ABCD is a rectangle.
Proof: In ∆ACB and ∆BDA,
AC = BD | Given
AB = BA | Common
BC = AD
| Opposite sides of || gm ABCD
∴ ∆ACB ≅ ∆BDA
| SSS Congruence Rule
∴ ∠ABC = ∠BAD ...(1) C.P.C.T.
Again, ∵ AD || BC
| Opp. sides of || gm ABCD and transversal AB intersects them.
∴ ∠BAD + ∠ABC = 180° ...(2)
| Sum of consecutive interior angles on the same side of a transversal is 180°
From (1) and (2),
∠BAD = ∠ABC = 90°
∴ ∠A = 90°
∴ || gm ABCD is a rectangle.
Long Answer TypeShort Answer TypeDiagonal AC of a parallelogram ABCD bisects ∠A (see figure). Show that:
(i) it bisects ∠C also
(ii) ABCD is a rhombus.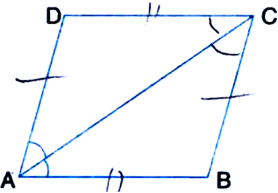
Long Answer Type
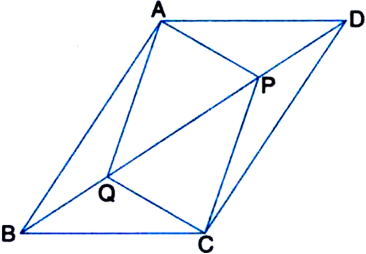
(i) ∆APD ≅ ∆CQB
(ii) AP = CQ
(iii) ∆AQB ≅ ∆CPD
(iv) AQ = CP
(v) APCQ is a parallelogram.
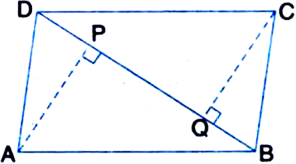
Short Answer TypeABCD is a parallelogram and AP and CQ are perpendiculars from vertices A and C on diagonal BD respectively (see figure). Show that:
(i) ∆APB ≅ ∆CQD
(ii) AP = CQ.
Switch
