Long Answer Type
Long Answer Type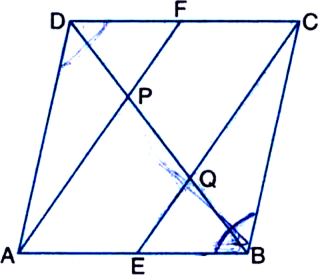
ABC is a triangle right angled at C. A line through the mid-point M of hypotenuse AB and parallel to BC intersects AC at D. Show that:
(i) D is the mid-point of AC (ii) MD ⊥ AC (iii) ![]()
 Short Answer Type
Short Answer TypeGiven: ABCD is a rhombus and AB is produced to E and F such that AE = AB = BF.
To Prove: ED ⊥ FC.
Proof: AB = BF | By construction
AB = BC
| ∵ ABCD is a rhombus
∴ BC = BF
∴ ∠1 = ∠2 ...(1)
| Angles opposite to equal sides of a ∆ are equal
In ∆BCF,
Ext. ∠3 = ∠1 + ∠2 = ∠1 + ∠1
| From(1)
= 2 ∠1 ...(2)
AB = AE | By construction
AB = AD
| ∵ ABCD is a rhombus
∴ AD = AE
∴ ∠5 = ∠6 ...(3)
| Angles opposite to equal sides of a ∆ are equal
In ∆ADE,
Ext. ∠4 = ∠5 + ∠6 = ∠5 + ∠5
| From (3)
= 2 ∠5 ...(4)
∵ AD || BC
and transversal AB intersects them
∴ ∠3 + ∠4 = 180°
| ∵ Consecutive interior angles on the same side of a transversal are supplementary
⇒ 2∠1 + 2∠5 = 180° | From (2) and (4)
⇒ ∠1 + ∠5 = 90° ...(5)
In ∆GEF,
∠1 + ∠5 + ∠EGF = 180°
| ∵ The sum of the three angles of a ∆ is 180°
⇒ 90° + ∠EGF = 180° | From (5)
⇒ ∠EGF = 90°
⇒ EG ⊥ GF
⇒ ED ≁ FC.
 Long Answer Type
Long Answer TypeD, E, F are respectively the midpoints of the sides BC, CA and AB of a triangle ABC. Show that:
(i) BDEF is a parallelogram
(ii) DFEC is a parallelogram.
 Short Answer Type
Short Answer Type
 Long Answer Type
Long Answer Type