Previous Year Papers

Download Solved Question Papers Free for Offline Practice and view Solutions Online.

Test Series

Take Zigya Full and Sectional Test Series. Time it out for real assessment and get your results instantly.

Test Yourself

Practice and master your preparation for a specific topic or chapter. Check you scores at the end of the test.

Quadrilaterals

Long Answer Type

41. In a parallelogram ABCD, E and Fare the mid-points of sides AB and CD respectively. (see figure). Show that the line segments AF and EC trisect the diagonal BD.

322 Views

42. Show that the line segments joining the mid-points of the opposite sides of a quadrilateral bisect each other.

333 Views

43.

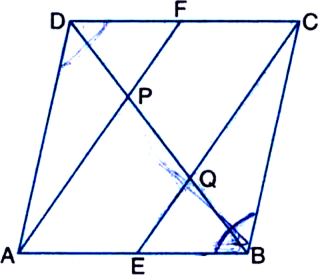
ABC is a triangle right angled at C. A line through the mid-point M of hypotenuse AB and parallel to BC intersects AC at D. Show that:

(i) D is the mid-point of AC (ii) MD ⊥ AC (iii) $CM space equals space MA space equals space 1 half AB.$

443 Views

Short Answer Type

44. In triangle ABC, points M and N on sides AB and AC respectively are taken so that

AM equals 1 fourth AB space and space AN equals 1 fourth AC comma space

Prove that MN =

1 fourth

113 Views

45. In triangle ABC, points M and N on sides AB and AC respectively are taken so that

AM equals 1 fourth AB space and space AN equals 1 fourth AC comma space Prove space that space MN equals 1 fourth

280 Views

46. ABCD is a rhombus and AB is produced to E and F such that AE = AB = BF. Prove that ED and FC are perpendicular to each other.

1943 Views

Long Answer Type

47. In ∆ABC, AD is the median through A and E is the mid-point of AD. BE is produced to meet AC in F. Prove that

AF equals 1 third AC

203 Views

48.

D, E, F are respectively the midpoints of the sides BC, CA and AB of a triangle ABC. Show that:
(i) BDEF is a parallelogram
(ii) DFEC is a parallelogram.

470 Views

Short Answer Type

49. In the figure ABCD is a parallelogram and E is the mid-point of side BC. DE and AB on producing meet at F. Prove that AF = 2AB.

233 Views

Long Answer Type

50. ABCD is a trapezium in which side AB is parallel to the side DC and E is the mid-point of side AD (see figure). If F is a point on the side BC such that the segment EF is parallel to the side DC, prove that F is the mid-point of BC and EF = $1 half$ (AB + DC).

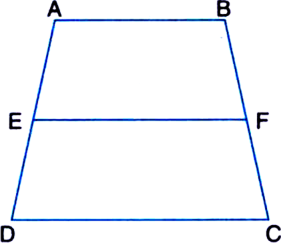
Given: ABCD is a trapezium in which side AB is parallel to the side DC and E is the mid-point of side AD. F is a point on the side BC such that the segment EF is parallel to the side DC.

To Prove: F is the mid-point of BC

and    $EF equals 1 half left parenthesis AB plus DC right parenthesis$

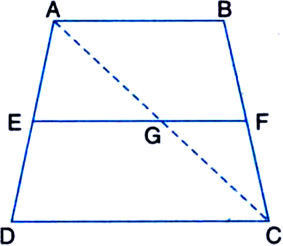
Given: ABCD is a trapezium in which side AB is parallel to the side D

Construction: Join AC to intersect EF at G.
Proof:
(i) In ∆ADC,
∵ E is the mid-point of AD and
EG || DC    | ∵ EF || DC
∴ G is the mid-point of AC
| by converse of mid-point theorem Again, In ∆CAB,
∵ G is the mid-point of AC | proved above
and    GF || DC    | ∵ EF || DC
∴ F is the mid-point of BC | by converse of
mid-point theorem
(ii) We shall prove that the line segment joining the mid-points of any two sides of a triangle is parallel to the third side and half of it.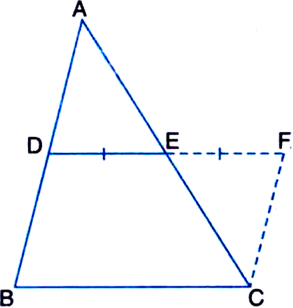

Given: ABCD is a trapezium in which side AB is parallel to the side D

Let ABC be a triangle in which D and E are the mid-points of the sides AB and AC respectively.
We produce DE to F such that DE = EF and join FC.
In ∆AED and ∆CEF,
AE = CE
| ∵ E is the mid-point of AC
∠AED = ∠CEF
| Vertically opposite angles
ED = EF    | by construction
∴ ∆AED ≅ ∆CEF
| SAS congruence rule
∴ AD = CF    |CPCT
and ∠ADE = ∠CFE    | CPCT
Now, ∵ D is the mid-point of AB
∴ AD = DB
But AD = CF    | Proved above
∴ DB = CF    ...(1)
Again, ∵ DF intersects AD and FC such that
∠ADE = ∠CFE    | Proved above
But these angles form a pair of equal alternate interior angles
∴ AD || FC
⇒ DB || FC    ...(2)
In view of (1) and (2),
DBCF is a parallelogram
| A quadrilateral is a parallelogram if one pair of its opposite sides is parallel and equal
∴ DF = BC
| Opposite sides of a parallelogram are equal
⇒ 2DE = BC    | by construction

$rightwards double arrow space space space DE equals 1 half BC$

Also, ∵ DBCF is a parallelogram
∴ DF || BC
| Opposite sides of a parallelogram are parallel
⇒ DE || BC
(iii) Using this result in ∆ADC,

$EG equals 1 half DC space space space space space space space space space space space space space space space space space space space space space space space space space.... left parenthesis 3 right parenthesis$

| ∵ E is the mid-point of AD and G is the mid-point of AC
and in ∆CAB,

$GF equals 1 half AB space space space space space space space space space space space space space space space space space space space space space... left parenthesis 4 right parenthesis$

| ∵ G is the mid-point of AC and F is the mid-point of BC
Adding (3) and (4), we get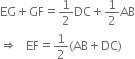

$EG plus GF equals 1 half DC plus 1 half AB rightwards double arrow space space space EF equals 1 half left parenthesis AB plus DC right parenthesis$