 Multiple Choice Questions
Multiple Choice QuestionsIf the altitude of a triangle are in arithmatic progression, then the sides of the triangles are in
AP
HP
GP
AGP
B.
HP
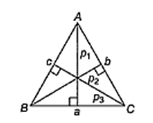
where a, b, c length of the sides of the tnangle and p1, p2, p3 are respectively altitudes
Give that p1, p2, p3 are in AP
 Match The Following
Match The FollowingThe correct matching of List-I from List-II is :
List- I List-II
(A) (i)
(B) (ii)
(C) if x > 1, then (iii)
(D) (iv)
(v)
(vi)
| A. A B C D | (i) (i) (iii) (iv) (v) |
| B. A B C D | (ii) (ii) (iii) (iv) (v) |
| C. A B C D | (iii) (iii) (ii) (iv) (v) |
| D. A B C D | (iv) (ii) (iii) (i) (v) |
 Multiple Choice Questions
Multiple Choice Questions