Previous Year Papers

Download Solved Question Papers Free for Offline Practice and view Solutions Online.

Test Series

Take Zigya Full and Sectional Test Series. Time it out for real assessment and get your results instantly.

Test Yourself

Practice and master your preparation for a specific topic or chapter. Check you scores at the end of the test.

Some Applications of Trigonometry

Short Answer Type

A circus artist is climbing a 20 m long rope, which is tightly stretched and tied from the top of a vertical pole to the ground. Find the height of the pole, if the angle made by the rope with the ground level is 30° (see Fig. 9.11)

Fig. 9.11

2146 Views

A tree breaks due to storm and the broken part bends so that the top of the tree touches the ground making an angle 30° with it. The distance between the foot of the tree to the point where the top touches the ground is 8 m. Find the height of the tree.

1331 Views

A contractor plans to install two slides for the children to play in a park. For the children below the age of 5 years, she prefers to have a slide whose top is at a height of 1.5 m, and is inclined at an angle of 30° to the ground, whereas for elder children, she wants to have a steep slide at a height of 3m, and inclined at an angle of 60° to the ground. What should be the length of the slide in each case?

884 Views

The angle of elevation of the top of a tower from a point on the ground, which is 30 m away from the foot of the tower, is 30°. Find the height of the tower.

1076 Views

A kite is flying at a height of 60 m above the ground. The string attached to the kite is temporarily tied to a point on the ground. The inclination of the string with the ground is 60°. Find the length of the string, assuming that there is no slack in the string.

1406 Views

Long Answer Type

6. A 1.5 m tall boy is standing at some distance from a 30 m tall building. The angle of elevation from his eyes to the top of the building increases from 30° from 60°, as he walks towards the building. Find the distance he walked towards the building.

590 Views

Short Answer Type

From a point on the ground, the angles of elevation of the bottom and the top of a transmission tower fixed at the top of a 20 m high building are 45° and 60° respectively. Find the height of the tower.

496 Views

Long Answer Type

8.
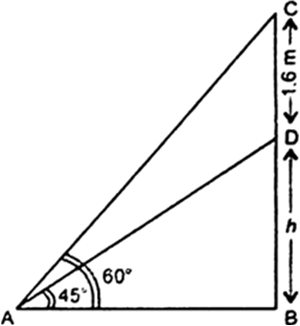
A statue, 1.6 m tall, stands on the top of a pedestal. From a point on the ground, the angle of elevation of the top of the statue is 60° and from the same point the angle of elevation of the top of the pedestal is 45°. Find the height of the pedestal.

Let height of the pedestal BD be h metres, and angle of elevation of C and D at a point A on the ground be 60° and 45° respectively.
It is also given that the height of the statue CD be 1.6 m
i.e., ∠CAB = 60°,
∠DAB = 45° and CD = 1.6m
In right triangle ABD, we have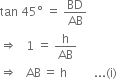

$tan space 45 degree space equals space BD over AB rightwards double arrow space space space 1 space equals space straight h over AB rightwards double arrow space space space AB thin space equals space straight h space space space space space space space space space... left parenthesis straight i right parenthesis$
In right triangle ABC, we have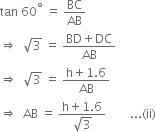

$tan space 60 to the power of degree space equals space BC over AB rightwards double arrow space space square root of 3 space equals space fraction numerator BD plus DC over denominator AB end fraction rightwards double arrow space space square root of 3 space equals space fraction numerator straight h plus 1.6 over denominator AB end fraction rightwards double arrow space space AB space equals space fraction numerator straight h plus 1.6 over denominator square root of 3 end fraction space space space space space space space space... left parenthesis ii right parenthesis$
Let height of the pedestal BD be h metres, and angle of elevation of
Comparing (i) and (ii), we get
$Let height of the pedestal BD be h metres, and angle of elevation of$ $straight h space equals space fraction numerator straight h space plus 1.6 over denominator square root of 3 end fraction rightwards double arrow space space space square root of 3 space end root space straight h space equals space straight h space plus space 1.6 rightwards double arrow space space space square root of 3 space straight h space minus space straight h space equals space 1.6 rightwards double arrow space space space straight h left parenthesis square root of 3 minus 1 right parenthesis space equals space 1.6 rightwards double arrow space space space straight h space equals space fraction numerator 1.6 over denominator square root of 3 minus 1 end fraction straight x fraction numerator square root of 3 plus 1 over denominator square root of 3 plus 1 end fraction rightwards double arrow space space space straight h space equals space fraction numerator 1.6 left parenthesis square root of 3 plus 1 right parenthesis over denominator 3 minus 1 end fraction rightwards double arrow space space space straight h space equals space 0.8 open parentheses square root of 3 plus 1 close parentheses$
Hence, the height of pedestal $equals space 0.8 space left parenthesis square root of 3 plus 1 right parenthesis straight m$

662 Views

The angle of elevation of the top of a building from the foot of the tower is 30° and the angle of elevation of the top of the tower from the foot of the building is 60°. If the tower is 50 m high, find the height of the building.

228 Views

Short Answer Type

10.

Two poles of equal heights are standing opposite each other on either side of the road, which is 80 m wide. From a point between them on the road, the angles of elevation of the top of the poles are 60° and 30°, respectively. Find the height of the poles and the distances of the point from the poles.

388 Views