 Short Answer Type
Short Answer Type
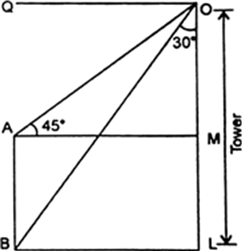
 Long Answer Type
Long Answer TypeLet AB be the tower of height h metres, D and C are two points on the horizontal line, which are at distances a and b metres respectively from the base of the tower. It is also given that the angles of elevation of the top of a tower from two points D and C be complementary i.e.,
∠ADB = Ս then ∠ACB = (90 -ө)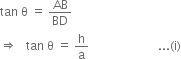
In right triangle ADB, we have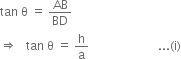
In right triangle ACB, we have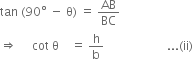
Multiplying (i) and (ii), we get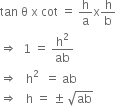
But height can't be negative.![]()
Hence the height of the tower is ![]() mts.
mts.
