Previous Year Papers

Download Solved Question Papers Free for Offline Practice and view Solutions Online.

Test Series

Take Zigya Full and Sectional Test Series. Time it out for real assessment and get your results instantly.

Test Yourself

Practice and master your preparation for a specific topic or chapter. Check you scores at the end of the test.

Some Applications of Trigonometry

Long Answer Type

31. The angles of depression of the top and the bottom of a 9 m high building from the top of a tower are 30° and 60° respectively. Find the height of the tower and the distance between the building and the tower.

236 Views

32. From the top of a building 60 m high the angles of depression of the top and the bottom of tower are observed to be 30° and 60°. Find the height of the tower.

2320 Views

33. As observed from the top of n lighthouse, 100 m high above sea level, the angle of depression of a ship sailing directly towards it, changes from 30° to 60°. Determine the distance travelled by the ship during the period of observation.

2691 Views

34. From the top of a hill, the angles of depression of two consecutive kilometre stones due east are found to be 30° and 45° respectively. Find the height of the hill.

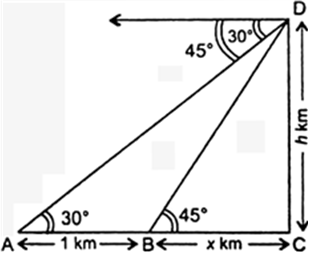
Let CD be the hill of height h km. Let A and B be two stones due east of the hill at a distance of 1 km. from each other. It is also given that the angles of depression of. stones A and B from the top of a hill be 30° and 45° respectively.
Let BC = x km
In right triangle BCD, we have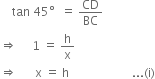

$space space space space tan space 45 degree space space equals space CD over BC rightwards double arrow space space space space space 1 space equals space straight h over straight x rightwards double arrow space space space space space space straight x space equals space straight h space space space space space space space space space space space space space space space space space space space space space... left parenthesis straight i right parenthesis$
In right triangle ACD, we have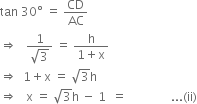

$tan space 30 degree space equals space CD over AC rightwards double arrow space space space fraction numerator 1 over denominator square root of 3 end fraction space equals space fraction numerator straight h over denominator 1 plus straight x end fraction rightwards double arrow space space 1 plus straight x space equals space square root of 3 straight h rightwards double arrow space space space straight x space equals space square root of 3 straight h space minus space 1 space space equals space space space space space space space space space space space space space space space... left parenthesis ii right parenthesis$
Comparing (i) and (ii), we get

$straight h space equals space square root of 3 space straight h space minus space 1 rightwards double arrow space square root of 3 straight h end root minus straight h space equals space 1 rightwards double arrow space straight h open parentheses square root of 3 minus 1 close parentheses space equals space 1 rightwards double arrow space straight h space equals space fraction numerator 1 over denominator square root of 3 minus 1 end fraction straight x fraction numerator square root of 3 plus 1 over denominator square root of 3 plus 1 end fraction rightwards double arrow space space straight h space equals space fraction numerator square root of 3 plus 1 over denominator left parenthesis square root of 3 right parenthesis squared minus left parenthesis 1 right parenthesis squared end fraction rightwards double arrow space space space space equals space space fraction numerator square root of 3 plus 1 over denominator 3 minus 1 end fraction rightwards double arrow space space space fraction numerator square root of 3 plus 1 over denominator 2 end fraction equals fraction numerator 1.732 plus 1 over denominator 2 end fraction equals fraction numerator 2.732 over denominator 2 end fraction equals space space 1.365 space km.$
Hence, the height of hill is 1.365.

1388 Views

35. An aeroplane when flying at a height of 3000 m from the ground passes vertically above another aeroplane at an instant when the angles of elevation of the two planes from the same point on the ground are 60° and 45° respectively. Find the vertical distance between the aeroplane at that instant.

515 Views

36. A 7 m long flagstaff is fixed on the top of a tower on the horizontal plane. From a point on the ground, the angles of elevation of the top and bottom of the flagstaff are 45° and 30° respectively. Find the height of the tower.

3110 Views

37. A person standing on the bank of a river observes that the angle of elevation of the top of a tree standing on the opposite bank is 60°. When he moves 40 metres away from the bank, he finds the angle of elevation to be 30°. Find the height of the tree and the width of the river.

1375 Views

38. From a point 100 m above a lake, the angle of elevation of a stationary helicopter is 30° and the angle of depression of reflection of the helicopter in the lake is 60°. Find the height of the helicopter.

2837 Views

39. A vertical tower stands on a horizontal plane and is surmounted by a vertical flagstaff of height y. At a point on the plane, the angles of elevation of the bottom and the top of the flagstaff are a and fi respectively. Prove that the height of the tower is

fraction numerator straight y space tan space straight alpha over denominator tan space straight beta space minus space tan space straight alpha end fraction.

1284 Views

Short Answer Type

40. If the angles of elevation of a cloud from a point h metres above a lake is α and the angle of depression of its reflection in the lake is β, prove that the height of the cloud is

straight h fraction numerator left parenthesis tan space straight beta space plus space tan space straight alpha right parenthesis over denominator tan space straight beta space minus space tan space straight alpha end fraction

218 Views