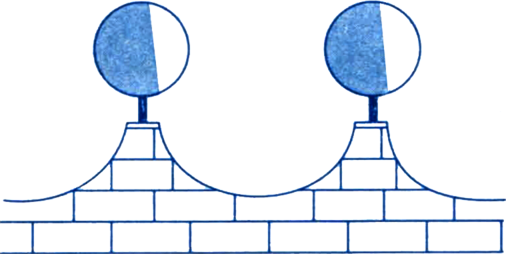
Short Answer TypeThe front compound wall of a house is decorated by wooden spheres of diameter 21 cm, placed on small supports as shown in figure. Eight such spheres are used for this purpose, and are to be painted silver. Each support is a cylinder of radius 1.5 cm and height 7 cm and is to be painted black. Find the cost of paint required if silver paint costs 25 paise per cm2 and black paint costs 5 paise per cm2.
Long Answer TypeFind the mean of each of the following distributions :
(i)
|
xi |
10 |
15 |
20 |
25 |
30 |
35 |
40 |
Total |
|
fi |
4 |
6 |
8 |
18 |
6 |
5 |
3 |
50 |
(ii)
|
xi |
12 |
13 |
14 |
15 |
16 |
17 |
18 |
Total |
|
fi |
1 |
3 |
4 |
8 |
10 |
3 |
1 |
30 |
(iii)
|
xi |
50 |
75 |
100 |
125 |
150 |
175 |
200 |
Total |
|
fi |
12 |
18 |
50 |
70 |
25 |
15 |
10 |
200 |
Short Answer TypeThe following data have been overanged in ascending order. If the median of these data is 63, then find the value of x : 29, 32, 48, 50, x, x + 2, 72, 78, 84, 95
Let r cm be the radius of the cylinder and h cm be the height of the cylinder, then
r = 7 cm,
and h = (13–7) cm
= 6 cm.
Let r1 cm be the radius of the hemisphere, then
r1 = 7 cm
Now,
the inner curved surface area of the vessel
= C,S.A of hemisphere
+ C,S.A of cylinder
= (2πr12 + 2 π rh) cm2
= (2 π r2 + 2 π rh) cm2 [∵ r1 = r]
= [2 π r (r + h)] cm2
= (44 x 13) cm2
= 572 cm2.
Switch
