Short Answer TypeFind the length and foot of the perpendicular drawn from the point (3, 4, 5) on the line 
Long Answer Type
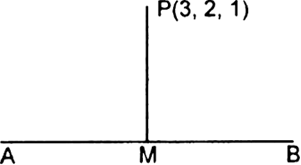
Any point M on this line is
(5 r + 4, 3 r + 2, 4 r + 3)
Let this point M be the foot of perpendicular from P (3, 2, 1) on AB.
Direction-ratios of PM are
5 r + 4 – 3, 3 r + 2 – 2, 4 r + 3 – 1 i.e. 5 r + 1, 3 r, 4 r + 2
Direction-ratios of AB are 5, 3, 4
Since PM ⊥ AB
∴ 5 (5 r + 1) + 3 (3 r) + 4 (4 + 2) = 0.
∴ 25 r + 5 + 9 r + 16 r + 8 = 0 ⇒ 50 r = – 13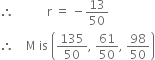
∴ required length of perpendicular = PM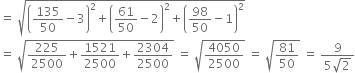
Short Answer Type
Long Answer TypeShort Answer Type
Switch
