 Short Answer Type
Short Answer Type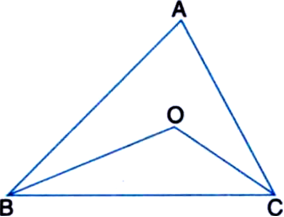
 Long Answer Type
Long Answer Type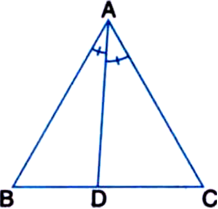
|∵ The sum of the lengths of any two sides of a triangle is greater than the length of the third side
In ∆OQR,
OQ + OR > QR ...(2)
| ∵ The sum of the lengths of any two sides of a triangle is greater than the length of the third
side
In AORP,
OR + OP > RP ...(3)
| ∵ The sum of the lengths of any two sides of a triangle is greater than the length of the third side
From (1), (2) and (3), we get,
(OP + OQ) + (OQ + OR) + (OR + OP) > (PQ + QR + RP)
⇒ 2(OP + OQ + OR) > (PQ + QR + RP)![]()
 Short Answer Type
Short Answer Type
 Multiple Choice Questions
Multiple Choice Questions


