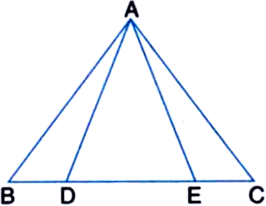
Short Answer Type
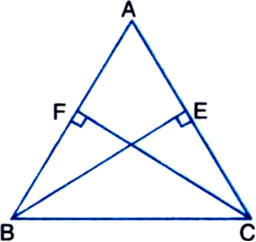
(i) ∆ABE ≅ ∆ACF
(ii) AB = AC, i.e., ∆ABC is an isosceles triangle.
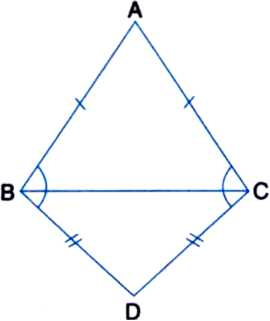
Long Answer Type∆ABC is an isosceles triangle in which AB = AC. Side BA is produced to D such that AD = AB (see figure). Show that ∆BCD is a right angle.
Short Answer TypeLong Answer TypeABC is a triangle in which ∠B = 2∠C. D is a point on side BC such that AD bisects ∠BAC and AB = CD. Prove that ∠BAC = 72°.
[Hint. Take a point P on AC such that BP bisects ∠B. Join P and D.]
Construction: Take a point P on AC such that BP bisects ∠B. Join P and D.
Proof: In ∆ABC,
∵ BP bisects ∠ABC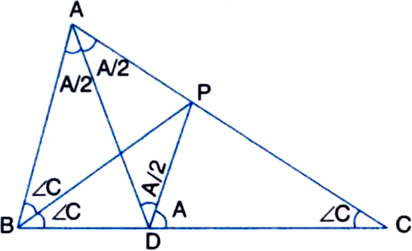
In APBC,
∴ ∠PBC = ∠PCB (= ∠C)
∴ PB = PC ...(2)
| Sides opposite to equal angles of∆PBC In ∆APB and ∆DPC,
AB = CD | Given
PB = PC | From (2)
∠ABP = ∠DCP (= ∠C)
∴ ∆APB ≅ ∆DPC | SAS Axiom
∴ ∠BAP = ∠CDP (= ∠A) ...(3)
| C.P.C.T.
and AP = DP ...(4) | C.P.C.T.
In ∆APD,
∵ AP = DP | From (4)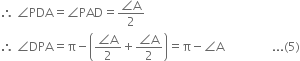
Again from ∆DPC,
∠DPC = π - (∠A + ∠C)
∴ ∠DPA = π - ∠DPC = π - {π - (∠A + ∠C)} = ∠A + ∠C ...(6)
From (5) and (6),
π - A = ∠A + ∠C ⇒ 2∠A + ∠C = π ...(7)
Again,
∠A + ∠B + ∠C = π
| ∵ The sum of three angles of ∆ABC = π ⇒ ∠A + 2∠C + ∠C = π | ∵ ∠B = 2∠C
⇒ ∠A + 3∠C = π ...(8)
Multiplying (7) by 3, we get
6∠A + 3∠C = 3π ...(9)
Subtracting (8) from (9), we get
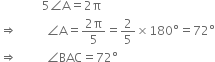
Short Answer Type
Switch
