Short Answer Type
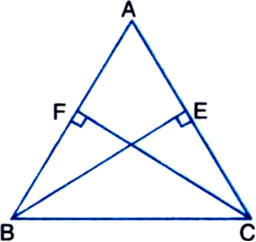
(i)    ∆ABE ≅ ∆ACF
(ii)    AB = AC, i.e., ∆ABC is an isosceles triangle.
Long Answer Type∆ABC is an isosceles triangle in which AB = AC. Side BA is produced to D such that AD = AB (see figure). Show that ∆BCD is a right angle.Â
Short Answer TypeLong Answer TypeABC is a triangle in which ∠B = 2∠C. D is a point on side BC such that AD bisects ∠BAC and AB = CD. Prove that ∠BAC = 72°.
[Hint. Take a point P on AC such that BP bisects ∠B. Join P and D.]
Short Answer TypeIn ∆OAC and ∆ODB,
OA = OD Â Â Â | Given
OB = OC Â Â Â | Given
∠AOC = ∠DOB
| Vertically Opposite Angles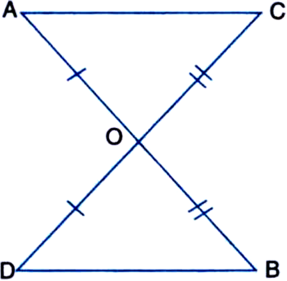
∴ ∆OAC ≅ ∆ODB    | SAS Axiom
∴ AC = BD    | C.P.C.T.
Also, ∠OAC = ∠ODB    | C.P.C.T.
and ∠OCA = ∠OBD    | C.P.C.T.
Thus ∠OAC may not be equal to ∠OBD and therefore, AC may not be parallel to BD
However, if OA = OC, then ∠OAC = ∠OCA
| Angles opposite to equal sides of ∆OAC But ∠OAC = ∠ODB
∴ ∠OCA = ∠ODB
But these angles form a pair of equal alternate angles
∴ AC || BD.
Switch
