 Multiple Choice Questions
Multiple Choice QuestionsThe area of the figure bounded by the curves y = cos(x) and y = sin(x) and the coordinate x = 0 and x =
The area enclosed between the parabola y = x2 - x + 2 and the line y = x + 2 in sq unit equals :
The area of the region bounded by y = 2x - x2 and the x-axis is
B.
Given curve is
y = 2x - x2
or (x - 1)2 = (y - 1)
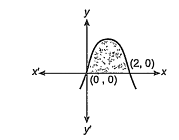
The curve cut the x-axis at (0, 0) and (2, 0).
The area bounded between the parabola y2 = 4x and the line y = 2x - 4 is equal to
9 sq unit
15 sq unit
