 Multiple Choice Questions
Multiple Choice QuestionsIf the vertex of the conic y - 4y = 4x - 4a always lies between the straight lines x + y = 3 and 2x + 2y - 1 = 0, then
2 < a < 4
0 < a < 2
The locus of the mid-points of chords of the circle x2 + y2 = 1, which subtends a right angle at the origin, is
x2 + y2 =
xy = 0
x2 - y2 = 0
The points of the ellipse 16x2 + 9y2 = 400 at which the ordinate decreases at the same rate at which the abscissa increases is/are given by
If the parabola x2 = ay makes an intercept of length units on the line y - 2x = 1, then a is equal to
1
- 2
- 1
2
If the vertex of the conic y - 4y = 4x - 4a always lies between the straight lines x + y = 3 and 2x + 2y - 1 = 0, then
2 < a < 4
0 < a < 2
If the point lies in the region between the lines x + y = 2 and x - y = 2 containing the origin, then 0 lies in
C.
Given that will lie on the circle (from the given figure). Since, point lies on the region containing origin.
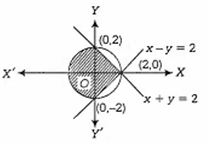
So, point will be on the shaded region.
Let 16x2 - 3y - 32x - 12y = 44 represents a hyperbola. Then,
length of the transverse axis is
length of each latusrectum is
eccentricity is
equation of a directrix is x =
If the straight line (a - 1)x - by + 4 = 0 is normal to the hyperbola xy = 1, then which of the following does not hold?
a > 1, b > 0
a > 1, b < 0
a < 1, b < 0
a < 1, b > 0
