 Multiple Choice Questions
Multiple Choice QuestionsIf f(x) = , then f is
continuous everywhere
discontinuous everywhere
continuous only at x = 0
continuous at all rational numbers
C.
continuous only at x = 0
If f(x) = . Then, which of the following is true ?
f(x) is not differentiable at x = a
f(x) is discontinuous at x = a
f(x) is continuous for all x < a
f(x) is differentiable for all x a
If f(x) is a function such that f"(a) + f'(a) = 0 and g(x) =[f(x)]2 + [f'(x)]2 and g(3) = 8, then g(8) is equal to
0
3
5
8
If f(x) = x3 and g(x) = x3 - 4x in - 2 , then consider the statements
(i) f(x) and g(x) satisfy mean value theorem.
(ii) f(x) and g(x) both satisfy Rolle's theorem.
(iii) Only g(x) satisfies Rolle's theorem.
Of these statements.
(i) and (ii) are correct
only (i) is correct
None is correct
(i) and (iii) are correct
The function represented by the following graph is
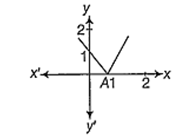
continuous but not differentiable at x = 1
differentiable but not continuous at x = 1
continuous and differentiable at x = 1
neither continuous nor differentiable at x = 1
