 Multiple Choice Questions
Multiple Choice QuestionsIf the pair of lines x - 16pxy - y2 = 0 and x2 - 16qxy - y = 0 are such that each pair bisects the angle between the other pair, then pq =
The equation of the pair of lines through the point (2, 1) and perpendicular to the pair of lines 4xy + 2x + 6y + 3 = 0 is
xy - x - 2y + 2 = 0
xy + x - 2y - 2 = 0
xy + x + 2y - 6 = 0
xy - x + 2y - 2 = 0
The point to which the origin is to be shifted to remove the first degree terms from the equation 2x2 + 4xy - 6y2 + 2x + 8y + 1 = 0 is
The figure formed by the pairs of lines
and
6x2 + 13xy + 6y + 10x + 10y + 4 = 0, is a
Square
Parallelogram
Rhombus
Rectangle
Let P(h, k) be a point on the curve y = x2 + 7x + 2, nearest to the line, y = 3x – 3. Then the equation of the normal to the curve at P is :
x + 3y - 62 = 0
x + 3y + 26 = 0
x - 3y - 11 = 0
x - 3y + 22 = 0
The Plane which bisects the line joining the points (4, – 2, 3) and (2, 4, – 1) at right angles also passes through the point :
(0, - 1, 1)
(4, 0, - 1)
(4, 0, 1)
(0, 1, - 1)
B.
(4, 0, - 1)
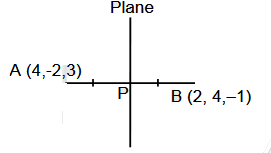
Mid point P = (3, 1, 1)
Normal of plane is along the line AB.
D.R.’s of normal = 4 – 2, – 2 – 4, 3 – ( – 1) = 2, – 6, 4 = 1, – 3, 2
Plane 1(x – 3) – 3(y – 1) + 2(z – 1) = 0
x – 3y + 2z – 2 = 0
The angle of elevation of a cloud C from a point P, 200 m above a still take is 30°. If the angle of depression of the image of C in the lake from the point P is 60°, then PC (in m) is equal to
100
400
