${\cos }^2\left(76°\right) + {\cos }^2\left(16°\right) - \cos \left(76°\right)\cos \left(16°\right) \mathrm{is} \mathrm{equal} \mathrm{to}$

• 0

• $\frac{1}{2}$

• $-\frac{1}{ 2}$

• $\frac{3}{4}$

$\sinh \left(\mathrm{ix}\right) \mathrm{is} \mathrm{equal} \mathrm{to}$

• $\mathrm{isin}\left(\mathrm{x}\right)$

• $\sin \left(\mathrm{ix}\right)$

• $- \mathrm{isin}\left(\mathrm{x}\right)$

• $\mathrm{isin}\left(\mathrm{ix}\right)$

The perimeter of a triangle is 16cm, one of the sides is of length 6cm. If the area of the triangle is 12 sq cm. Then the triangle is

• right angled

• isosceles

• equilateral

• scalene

If $∆$ABC is right angled at A, then r₂ + r₃ is equal to

• r₁ - r

• r₁ + r

• r - r₁

• R

If in $∆\mathrm{ABC}$, r₁ < r₂ < r₃, then :

• a < b < c

• a > b > c

• b > a < c

• a < c < b

In a $∆\mathrm{ABC}$, if 3a = b + c, then $\cot \left(\frac{\mathrm{B}}{2}\right)\cot \left(\frac{\mathrm{C}}{2}\right)$ is equal to :

• 1

• 2

• 3

• 4

If in a triangle, if b = 20, c = 21 and $\sin \left(\mathrm{A}\right) = \frac{3}{5}$, then a is equal to

• 12

• 13

• 14

• 15

198.

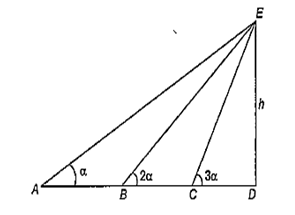
A tower subtends angles $\mathrm{\alpha }, 2\mathrm{\alpha } \mathrm{and} 3\mathrm{\alpha }$ respectively at points A, B and C, all lying on a horizontal line through the foot of the tower,then $\frac{\mathrm{AB}}{\mathrm{BC}}$ is equal to :

• $\frac{\sin \left(3\mathrm{\alpha }\right)}{\sin \left(2\mathrm{\alpha }\right)}$

• $1 + 2\cos \left(2\mathrm{\alpha }\right)$

• $2\cos \left(2\mathrm{\alpha }\right)$

• $\frac{\sin \left(2\mathrm{\alpha }\right)}{\sin \left(\mathrm{\alpha }\right)}$

The co-ordinate axes are rotated through an angle 135°. If the co-ordinates of a point P inthe new system are known to be (4, - 3), then the co-ordinates of P in the original system are :

• $\left(\frac{1}{\sqrt{2}}, \frac{7}{\sqrt{2}}\right)$

• $\left(\frac{1}{\sqrt{2}}, - \frac{7}{\sqrt{2}}\right)$

• $\left(- \frac{1}{\sqrt{2}}, - \frac{7}{\sqrt{2}}\right)$

• $\left(- \frac{1}{\sqrt{2}}, \frac{7}{\sqrt{2}}\right)$

The angle between the curves y = sin(x) and y = cos(x) is

• ${\tan }^{-1}\left(2\sqrt{2}\right)$

• ${\tan }^{-1}\left(3\sqrt{2}\right)$

• ${\tan }^{-1}\left(3\sqrt{3}\right)$

• ${\tan }^{-1}\left(5\sqrt{2}\right)$