An ideal monoatomic gas is taken around the cycle ABCDA as shown in the PV diagram. The work done during the cycle is given by

• $\frac{1}{2} \mathrm{PV}$

• PV

• 2 PV

• 4 PV

310 J of heat is required to raise the temperature of 2 moles of an ideal gas at constant pressure from 25°C to 35°C. The amount of heat required to raise the temperature of the gas through the same range at constant volume is

• 384 J

• 144 J

• 276 J

• 452 J

An ideal gas is taken via path ABCA as shown in figure. The net work done in the whole cycle is

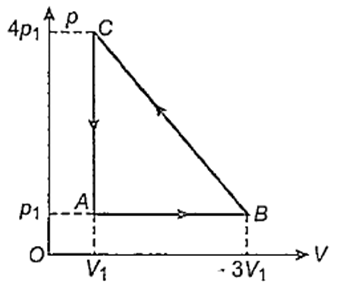
• 3p₁V₁

• − 3p₁V₁

• 6p₁V₁

• Zero

A graph of pressure versus volume for an ideal gas for different processes is as shown. In the graph curve OC represents

• isochoric process

• isothermal process

• isobaric process

• adiabatic process

The temperature of a gas contained in a closed vessel of constant volume increases by 1°C when the pressure of the gas is increased by 1%. The initial temperature of the gas is

• 100 K

• 273°C

• 100°C

• 200 K

The mean energy of a molecule of an ideal gas is

• $\frac{1}{2} \mathrm{KT}$

• 2 KT

• KT

• $\frac{3}{2} \mathrm{KT}$

The molecules of a given mass of a gas have r.m.s. velocity of 200 m/s at 27^o C and 1.0 x 10⁵ Nm^-2 pressure. When the temperature and pressure of the gas are respectively, 127^_o C and 0.05 x 10⁵  Nm^-2, the RMS velocity of its molecules in m/s is,

38.

The mean free path of molecules of a gas, (radius r) is inversely proportional to,

• r³

• r²

• r

• r

In the given (V-T )diagram, what is the relation between pressures p₁ and p₂?

• p₂ =p₁

• p₂>p₁

• p₂<p₁

• p₂<p₁

Two vessels separately contain two ideal gases A and B the same temperature, the pressure of A being twice that of B. Under such conditions, the density of A is found to be 1.5 times the density of B. The ratio of molecular weight of A and B is

• 2/3

• 3/4

• 2

• 2