 Multiple Choice Questions
Multiple Choice QuestionsThe magnetic field at the centre of a current carrying loop of radius 0.1 m is times that at a point along its axis. The distance of this point from the centre of the loop is
0.1 m
0.2 m
0.05 m
0.25 m
A wire carrying currenti is shaped as shown. Section AB is a quarter circle of radius r. The magnetic field is directed

perpendicular to the plane of the paper and directed into the paper
at an angle to the plane of the paper
along the bisector of the angle ACB away from AB
along the bisector of ACB towards AB
The wire loop formed by joining two semicircular sections of radii R1 and R2 and centre C, carries a current I as shown. The magnetic field at C has a magnitude

A particle of unit mass and specific charge s is thrown from the wall perpendicularly to a wall at a distance d from the wall with speed 'v'. The minimum magnetic field produced so that the particle does not touch the wall, is:
A long straight wire of radius a carries a steady current I. The current is uniformly distributed over its cross-section. The ratio of the magnetic fields B and B' at radial distances 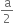 and 2a respectively, from the axis of the wire is,
and 2a respectively, from the axis of the wire is,
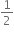
1
4
4
B.
1
b) Consider two amperian loops of radius a/2 and 2a as shown in the diagram.
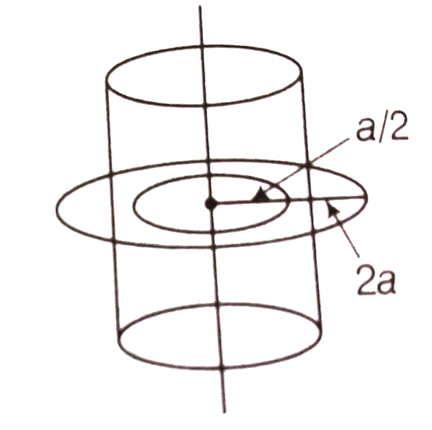


 (2a) =
(2a) = 
 , at a distance 2a from the axis of the wire.
, at a distance 2a from the axis of the wire.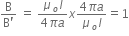
A magnetic needle suspended parallel to a magnetic field requires 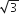 J of work to turn it through 60o. The torque needed to maintain the needle in this position will be
J of work to turn it through 60o. The torque needed to maintain the needle in this position will be
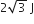
3 J


The ratio of amplitude of magnetic field to the amplitude of electric field for an electromagnetic wave propagating in vacuum is equal to
the speed of light in vacuum
the reciprocal of the speed of light in vacuum
the ratio of magnetic permeability to the electric susceptibility of vacuum
the ratio of magnetic permeability to the electric susceptibility of vacuum
Two similar coils of radius R are lying concentrically with their planes at right angles to each other. The currents flowing in them are I and 2I, respectively. The resultant magnetic field induction at the centre will be
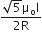
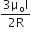
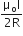
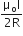
An electric dipole of moment p is placed in an electric field of intensity E. The dipole acquires a position such that the axis of the dipole makes an angle θ with the direction of the field. Assuming that the potential energy of the dipole to be zero when θ =90o, the torque and the potential energy of the dipole will respectively be
pE sin θ, pE cos θ
pE sin θ,-2pE cos θ
pE sin θ, 2 pE cos θ
pE sin θ, 2 pE cos θ
A wire loop is rotated in a magnetic field. The frequency of change of direction of the induced emf is
one per revolution
twice per revolution
four times per revolution
four times per revolution
