Previous Year Papers

Download Solved Question Papers Free for Offline Practice and view Solutions Online.

Test Series

Take Zigya Full and Sectional Test Series. Time it out for real assessment and get your results instantly.

Test Yourself

Practice and master your preparation for a specific topic or chapter. Check you scores at the end of the test.

Algebra

Multiple Choice Questions

21.

If $2 straight x plus 3 straight y space equals space 11 over 2$ and $xy space equals space 5 over 6$ , then the value of $8 straight x cubed plus 27 straight y cubed$ is

$583 over 4$
187
$671 over 8$
$671 over 8$

124 Views

22.

If $open curly brackets 1 half left parenthesis straight a minus straight b right parenthesis close curly brackets squared space plus space ab space equals space straight p left parenthesis straight a plus straight b right parenthesis squared comma$ then the value of p is (assume that $straight a not equal to negative straight b$ )

1
$1 half$
$1 fourth$
$1 fourth$

132 Views

23.
If 999x + 888y = 1332 and 888x + 999y = 555, then $straight x squared minus straight y squared$ is equal to

5

7

8

8

We have,
999x + 888y = 1332
and 888x + 999y = 555
On dividing both equation by 111,
we get
9x + 8y = 12 ...(i)
8x + 9y = 5 ...(ii)
On multiplying Eq. (i) by 9 and Eq. (ii) by 8, then subtract Eq.(ii) from Eq. (i), we get,
81x + 72y = 108
64x + 72y = -40
17x = 68
$straight x space equals space 68 over 17 space equals 4$
Put the value of x in Eq. (i) we get,
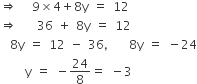
$rightwards double arrow space space space 9 cross times 4 plus 8 straight y space equals space 12 rightwards double arrow space space space space 36 space plus space 8 straight y space equals space 12 space space 8 straight y space equals space 12 space minus space 36 comma space space space 8 straight y space equals space minus 24 space space space space space straight y space equals space minus 24 over 8 equals space minus 3$
$therefore$ $straight x squared minus straight y squared space equals space left parenthesis 4 right parenthesis squared minus left parenthesis 3 right parenthesis squared space equals space 16 minus 9 space equals space 7$

626 Views

24.

If $straight x to the power of 4 plus 1 over straight x to the power of 4 space equals space 119$ , then the value of $straight x cubed minus 1 over straight x cubed$

36
-36
$plus-or-minus 36$
$plus-or-minus 36$

163 Views

25.

A man loses $20 1 half percent sign$ of his money and after spending 80% of the remainder, he is left with ₹ 159. How much did he have at first?

₹ 500
₹ 800
₹ 1000
₹ 1000

152 Views

26.

If $straight x space equals space 1 plus square root of 2 plus square root of 3 comma$ then the value of $straight x squared minus 2 straight x minus 4$ is:

$square root of 6$
$2 square root of 3$
$3 square root of 2$
$3 square root of 2$

186 Views

27.

If $fraction numerator left parenthesis straight a plus straight b right parenthesis over denominator square root of ab end fraction space equals 2 over 1 comma$ then the value of $left parenthesis straight a minus straight b right parenthesis$ is:

1
0
-1
-1

172 Views

28.

If $straight x plus 1 over straight x equals 5 comma$ then the value of $fraction numerator 6 straight x over denominator straight x squared plus straight x plus 1 end fraction$ is

151 Views

29.

If $straight a equals straight x to the power of 1 third end exponent straight y to the power of 1 third end exponent space plus space straight x to the power of negative 1 third end exponent straight y to the power of negative 1 third end exponent comma space then space space straight a cubed minus 3 ay minus straight x$ is equal to

$straight y squared over straight x$
$straight x squared over straight y$
$straight a squared over straight x$
$straight a squared over straight x$

172 Views

30.

If $straight a greater than straight b greater than 0 comma space space AB space equals space straight a minus straight b space cm comma space space space BC space equals space 2 square root of ab space cm comma space space then space angle ABC$ is