A telegraph post is bent at a point above the ground due to storm. Its top just touches the ground at a distance of 10√3 from its foot and makes an angle of 30° with the horizontal. Then height (in metres) of the telegraph post is

• 30

• 24

• 20

• 28

A vertical tower stands on a horizontal plane and is surmounted by a vertical flag staff of height h. At a point on the plane, the angle of elevation of the bottom of the flag staff is α and that of the top of the flag staff is β. Then the height of the tower is

23.

A man on the top of a tower, standing on the sea shore, finds that a boat coming towards him takes 10 minutes for the angle of depression to change from 30° to 60°. How soon the boat reach the sea-shore?

• 5 minutes

• 7 minutes

• 10 minutes

• 50 minutes

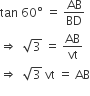
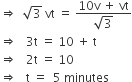
From the top of a cliff 100 metre high, the angles of depression of the top and bottom of a tower are 45° and 60° respectively. The height of the tower is

A person from the top of a hill observes a vehicle moving towards him at a uniform speed. It takes 10 minutes for the angle of depression to change from 45° to 60°. After this the time required by the vehicle to reach the bottom of the hill is

• 12 minutes 20 seconds

• 13 minutes

• 13 minutes 40 seconds

• 14 minutes 24 seconds

A man standing on the bank of a river observes that the angle of elevation of the top of a tree just on the opposite bank is 60°. But the angle of elevation is 30° from a point which is at a distance of  from the bank. Then the height of the tree

• 60 ft

• 45 ft

• 30 ft

• 30 ft

The shadow of a vertical tower on ground level increases by 10 m when the altitude of the sun changes from 45° to 30°. The height of the tower is

• 5( √3 + 1 ) m

• 10( √3 - 1 ) m

• 9 m

• 13 m

Two men are on opposite sides of a tower. They measure the angles of elevation of the top of the tower as 30° and 45° respectively. If the height of the tower is 50 m, the distance between the two men is 

• 136.5 m

• 50√3 m

• 100√3 m

• 135.5 m

The shadow of a tower when the angle of elevation of the sun is 45°, is found to be 10 m longer than when it was 60°. The height of the tower is

• 5( √3 - 1 ) m

• 5( √3 + 3 ) m

• 10( √3 - 1 ) m

• 10( √3 + 1 ) m

The angle of elevation of aeroplane from a point on the ground is 45°. After flying 15 sec, the elevation changes to 30°. If the aeroplane is flying at a height of 2500 m, then the speed of the aeroplane in km/hr is

• 600

• 600( √3 + 1 )

• 600√3

• 600( √3 - 1 )