The angles of elevation of the top of a tower from two points at a distance of 4 m and 9 m from the base of the tower and in the same straight line with it are complementary. The height of the tower is:

• 4 m

• 7 m

• 9 m

• 6 m

32.

A telegraph post is bent at a point above the ground. Its top just touches the ground at a distance of 8√3 from its foot and makes an angle of 30° with the horizontal. The height (in m) of the post is:

• 12

• 16

• 18

• 24

A pilot in an aeroplane at an altitude of 200 m observes two points lying on either side of a river. If the angles of depression of the two points be 45° and 60°, then the width of the river is

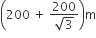
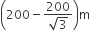
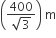
A boat is moving away from an observation tower. It makes an angle of depression of 60° with an observer's eye when at a distance of 50 m from the tower. After 8 sec, the angle of depression becomes 30°. By assuming that it is running in still water, the approximate speed of the boat is

• 33 km/hr

• 42 km/hr

• 45 km/hr

• 50 km/hr

The upper part of a tree broke at a certain height makes an angle of 60° with the ground at a distance of 10 m from its feet. The original height of the tree was

• 20√3 m

• 10√3 m

• 10(2 + √3) m

• 10(2 - √3) m

The angle of elevation of a ladder leaning against a wall is 60° and the foot of the ladder is 4.6 m away from the wall. The length of the ladder is

• 2.3 m

• 4.6 m

• 9.2 m

• 7.8 m

From a point on a bridge across the river, the angles of depression of the banks on opposite sides of the river are 30° and 45° respectively. If the bridge is at a height of 2.5 m from the banks, then the width of the river is (take ):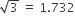

• 5.83 m

• 6.83 m

• 5.76 m

• 6.87 m

The length of shadow of a tower is √3 times that of its length. The angle of elevation of the sun is

• 45°

• 30°

• 60°

• None of these

A tower is 50 m high. Its shadow is x metres shorter than the sun's altitude is 45° than when it is 30°. The value of x in metres is

• 50√3

• 50( √3 - 1 )

• 50( √3 + 1 )

• 50

From the top of a 20 m high building, the angle of elevation of the top of a tower is 60° and the angle of depression of it's foot is at 45°, then the height of the tower is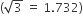 

• 45.46 m

• 45.64 m

• 54.64 m

• 54.46 m