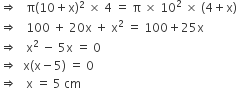Multiple Choice Questions
Multiple Choice QuestionsA solid cylinder has total surface area of 462 sq. cm. Curved surface area is  of its total surface area. The volume of the cylinder is
of its total surface area. The volume of the cylinder is
530 cm3
536 cm3
539 cm3
539 cm3
A cylinder and a cone have equal radii of their bases and equal heights. If their curved surface areas are in the ratio 8 : 5, the ratio of their radius and height is
1 : 2
1 : 3
2 : 3
2 : 3
A solid is hemispherical at the bottom and conical above. If the surface areas of the two parts are equal, then the ratio of radius and height of its conical part is
1 : 3
1 : 1
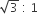
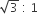
Base of a right prism is an equilateral triangle of side 6 cm. If the volume of the prism is  its height is
its height is
9 cm
10 cm
11 cm
11 cm
Base of a right pyramid is a square, length of diagonal of the base is 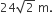 If the volume of the pyramid is 1728 cu.m, its height is
If the volume of the pyramid is 1728 cu.m, its height is
7 m
8 m
9 m
9 m
The ratio of radii of two right circular cylinders is 2 : 3 and their heights are in the ratio 5 : 4. The ratio of their curved surface area is
5 : 6
3 : 4
4 : 5
4 : 5
The height of a right circular cone and the radius of its circular base are respectively 9cm and 3 cm. The cone is cut by a plane parallel to its base so as to divide it into two parts. The volume of the frustum (i.e., the lower part) of the cone is 44 cubic cm. The radius of the upper circular surface of the frustrum (taking π = 22/7 ) is
∛12 cm
∛13 cm
∛6 cm
∛9 cm
The volume of a right circular cone is 1232 cm3 and its vertical height is 24 cm. Its curved surface area is
154 cm2
550 cm2
604 cm2
604 cm2
The volume of a solid hemisphere is 19404 cm3. Its total surface area is
4158 cm2
2858 cm2
1738 cm2
1738 cm2
The radius of a cylinder is 10 cm and height is 4 cm. The number of centimetres that may be added either to the radius or to the height to get the same increase in the volume of the cylinder is
5
4
25
25
A.
5
Let radius be increased by x cm.
∴ Volume of cylinder = 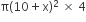 ...(i)
...(i)
Again, let height be increased by x cm.
∴ Volume of cylinder 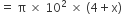 ...(ii)
...(ii)
According to the question
Equation (i) = Equation (ii)