 Multiple Choice Questions
Multiple Choice QuestionsA circle and a rectangle have the same perimeter. The sides of the rectangle are 18 cm and 26 cm. The area of the circle is 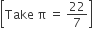
125 cm2
230 cm2
550 cm2
550 cm2
The area of a circle is increased by 22 cm2 when its radius is increased by 1 cm. The original radius of the circle is
3 cm
5 cm
7 cm
7 cm
256 sq. cm.
250 sq. cm.
128 sq. cm.
128 sq. cm.
The lengths of three medians of a triangle are 9cm, 12cm and 15 cm. The area (in sq. cm) of the triangle is
24
72
48
48
A playground is in the shape of a rectangle. A sum of ₹ 1,000 was spent to make the ground usable at the rate of 25 paise per sq. m. The breadth of the ground is 50 m. If the length of the ground is increased by 20 m, what will be the expenditure in rupees at the same rate per sq. m.?
1,250
1,000
1,500
1,500
A.
1,250
Area of rectangular field: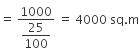
Breadth = 50 m (given)
New length of field = (80 + 20)m = 100 m
New area = 100 x 50 = 5000 sq.m.
∴ Required expenditure
In a triangle ABC, AB + BC = 12 Cm, BC + CA = 14 cm and CA + AB = 18 cm. Find the radius of the circle (in cm) which has the same perimeter as the triangle.
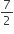
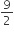
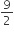
If the length of a rectangle is increased by 10% and its breadth is decreased by 10%, then its area
decrease by 1%
increases by 1%
decreases by 2%
decreases by 2%
A wire, when bent in the form of a square, encloses a region of area 121 cm2. If the same wire is bent into the form of a circle, then the area of the circle is 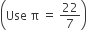
150 cm2
152 cm2
154 cm2
154 cm2
The ratio of the area of a sector of a circle to the area of the circle is 1 : 4. If the area of the circle is 154 cm2, the perimeter of the sector is
20 cm
25 cm
36 cm
36 cm
