Previous Year Papers

Download Solved Question Papers Free for Offline Practice and view Solutions Online.

Test Series

Take Zigya Full and Sectional Test Series. Time it out for real assessment and get your results instantly.

Test Yourself

Practice and master your preparation for a specific topic or chapter. Check you scores at the end of the test.

Trigonometry

Multiple Choice Questions

41.

a, b, c are the lengths of three sides of a triangle ABC. If a, b, c are related by the relation a² + b² + c² = ab + bc + ca, then the value of sin²A+ sin²B + sin²C is

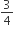
$3 over 4$
$fraction numerator 3 square root of 3 over denominator 2 end fraction$
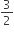
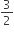
$3 over 2$
$3 over 2$

247 Views

42.

The value of $straight theta space left parenthesis 0 space less or equal than space straight theta space less or equal than space 90 degree right parenthesis$ satisfying $2 space sin squared straight theta space equals space 3 space cosθ$ is

$60 degree$
$30 degree$
$90 degree$
$90 degree$

166 Views

43.

If $tan space straight x space equals space sin space 45 degree. space cos space 45 degree space plus space sin space 30 degree$ then the value of x is

$30 degree$
$45 degree$
$60 degree$
$60 degree$

128 Views

44.

For any real values of θ, $fraction numerator square root of secθ space minus 1 end root over denominator square root of secθ space plus 1 end root end fraction space equals space ?$

$cot space straight theta space minus space cosec space straight theta$
$secθ space minus space tanθ$
$cosecθ space minus space cotθ$
$cosecθ space minus space cotθ$

250 Views

45.
If sin 3A = cos (A - 26°), where 3A is an acute angle then the value of A is

29°

26°

23°

23°

29°

sin 3A = cos (A - 26°).
⇒ cos (90° - 3A) = cos (A - 26°)
⇒ 90° - 3A = A - 26°
⇒ 90° + 26° = 3A + A
⇒ 4A = 116
$rightwards double arrow space space straight A space equals space 116 over 4 space equals space 29 degree$

178 Views

46.

Find the value of $sec squared straight theta space minus space fraction numerator sin squared straight theta space minus space 2 sin to the power of 4 straight theta over denominator 2 cos to the power of 4 straight theta space minus space cos squared straight theta end fraction$

1
2
-1
-1

153 Views

47.

If x = a(sinθ + cosθ), y = b(sinθ - cosθ) then the value of $straight x squared over straight a squared space plus space straight y squared over straight b squared$ is

202 Views

48.

If sin 5θ = cos 20° (0° < θ < 90°) then the value of θ is

4°
22°
10°
10°

140 Views

49.

ABCD is a rectangle of which AC is a diagonal. The value of is

183 Views

50.

In a Δ ABC, $angle straight B space equals space straight pi over 3 comma space space angle straight C space equals space straight pi over 4$ and D divides BC internally in the ratio 1 : 3 then $fraction numerator sin space angle BAD over denominator sin space angle CAD end fraction$ is equal to