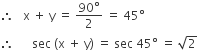Multiple Choice Questions
Multiple Choice QuestionsIf cos x = sin y and cot (x - 40°) = tan (50° - y), then the value of x and y are:
x = 70°, y = 20°
x = 75°, y = 15°
x = 85°, y = 5°
x = 80°, y = 10°
If sin θ + sin2θ = 1, then the value of cos12 θ + 3 cos 10 θ + 3 cos8 θ + cos6 θ - 1 is
0
1
-1
2
If x, y are positive acute angles x + y < 90° and sin (2x - 20°) = cos (2y + 20°), then the values of sec (x + y) is


1
0
A.

Given,
sin (2x - 20°) = cos (2y + 20°)
⟹ cos [90° - (2x - 20°)] = cos (2y + 20°)
⟹ 90° - 2x + 20° = 2y + 20°