CBSE
 Multiple Choice Questions
Multiple Choice Questions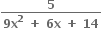 ની મહત્તમ કિંમત ..... છે.
ની મહત્તમ કિંમત ..... છે.


13
5
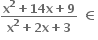 ....... મળે.
....... મળે.
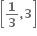
D.
[-5, 4]Tips: -
ધારો કે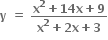
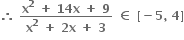
 ને ........ બીજ મળે.
ને ........ બીજ મળે.
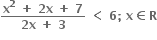 હોય, તો x ની ...... કિંમતો સત્ય બને.
હોય, તો x ની ...... કિંમતો સત્ય બને.
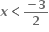 અથવા -1 < x < 11
અથવા -1 < x < 11
બે વાસ્તવિક
 નું x દ્વારા સમાધાન થતું હોય તો ની કિંમતો ........ હોઈ શકે. (x > 0)
નું x દ્વારા સમાધાન થતું હોય તો ની કિંમતો ........ હોઈ શકે. (x > 0) 4, -5
5, -4
4
5
 =...... થાય.
=...... થાય.
2/3
1/3
5
1/3
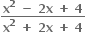 ની મહત્તમ ન્યુનતમ કિંમત અનુક્રમે ........ હોય.
ની મહત્તમ ન્યુનતમ કિંમત અનુક્રમે ........ હોય.
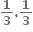
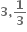
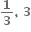
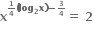 નાં બીજ .......... મળે.
નાં બીજ .......... મળે.
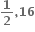
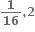
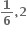
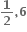
b/c સમગુણોત્તર શ્રેણીમાં હોય